Page 66 - Understanding Psychology

Transforming Scores
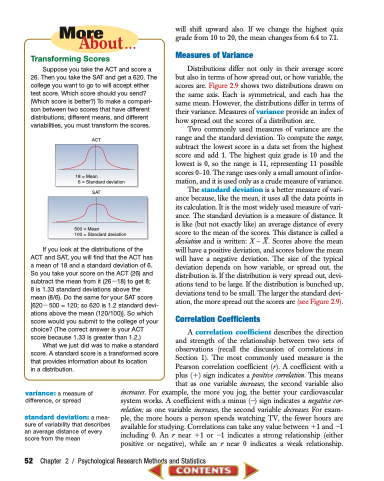
Suppose you take the ACT and score a 26. Then you take the SAT and get a 620. The college you want to go to will accept either test score. Which score should you send? (Which score is better?) To make a compari- son between two scores that have different distributions, different means, and different variabilities, you must transform the scores.
If you look at the distributions of the
ACT and SAT, you will find that the ACT has
a mean of 18 and a standard deviation of 6. So you take your score on the ACT (26) and subtract the mean from it (26􏰁18) to get 8;
8 is 1.33 standard deviations above the
mean (8/6). Do the same for your SAT score [620􏰁500 = 120; so 620 is 1.2 standard devi- ations above the mean (120/100)]. So which score would you submit to the college of your choice? (The correct answer is your ACT score because 1.33 is greater than 1.2.)
What we just did was to make a standard score. A standard score is a transformed score that provides information about its location
in a distribution.
will shift upward also. If we change the highest quiz grade from 10 to 20, the mean changes from 6.4 to 7.1.
Measures of Variance
Distributions differ not only in their average score but also in terms of how spread out, or how variable, the scores are. Figure 2.9 shows two distributions drawn on the same axis. Each is symmetrical, and each has the same mean. However, the distributions differ in terms of their variance. Measures of variance provide an index of how spread out the scores of a distribution are.
Two commonly used measures of variance are the range and the standard deviation. To compute the range, subtract the lowest score in a data set from the highest score and add 1. The highest quiz grade is 10 and the lowest is 0, so the range is 11, representing 11 possible scores 0–10. The range uses only a small amount of infor- mation, and it is used only as a crude measure of variance.
The standard deviation is a better measure of vari- ance because, like the mean, it uses all the data points in its calculation. It is the most widely used measure of vari- ance. The standard deviation is a measure of distance. It is like (but not exactly like) an average distance of every score to the mean of the scores. This distance is called a deviation and is written: X – X–. Scores above the mean will have a positive deviation, and scores below the mean will have a negative deviation. The size of the typical deviation depends on how variable, or spread out, the distribution is. If the distribution is very spread out, devi- ations tend to be large. If the distribution is bunched up, deviations tend to be small. The larger the standard devi- ation, the more spread out the scores are (see Figure 2.9).
Correlation Coefficients
A correlation coefficient describes the direction and strength of the relationship between two sets of observations (recall the discussion of correlations in Section 1). The most commonly used measure is the Pearson correlation coefficient (r). A coefficient with a plus (􏰀) sign indicates a positive correlation. This means that as one variable increases, the second variable also
ACT
18 = Mean
6 = Standard deviation
SAT
500 = Mean
100 = Standard deviation
variance: a measure of difference, or spread
standard deviation: a mea- sure of variability that describes an average distance of every score from the mean
increases. For example, the more you jog, the better your cardiovascular system works. A coefficient with a minus (􏰁) sign indicates a negative cor- relation; as one variable increases, the second variable decreases. For exam- ple, the more hours a person spends watching TV, the fewer hours are available for studying. Correlations can take any value between 􏰀1 and 􏰁1 including 0. An r near 􏰀1 or 􏰁1 indicates a strong relationship (either positive or negative), while an r near 0 indicates a weak relationship.
52 Chapter 2 / Psychological Research Methods and Statistics