Page 185 - Geosystems An Introduction to Physical Geography 4th Canadian Edition
P. 185
Chapter 6 atmospheric and Oceanic Circulations 149
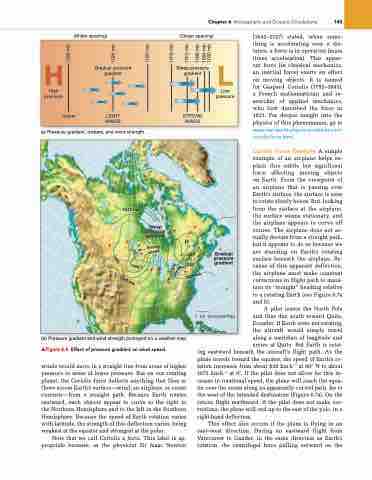
(Wider spacing)
(Closer spacing)
Steep pressure gradient
STRONG WINDS
(1643–1727) stated, when some thing is accelerating over a dis tance, a force is in operation (mass times acceleration). This appar ent force (in classical mechanics, an inertial force) exerts an effect on moving objects. It is named for Gaspard Coriolis (1792–1843), a French mathematician and re searcher of applied mechanics, who first described the force in 1831. For deeper insight into the physics of this phenomenon, go to www.real-world-physics-problems.com/ coriolis-force.html.
Coriolis Force Example A simple example of an airplane helps ex plain this subtle but significant force affecting moving objects on Earth. From the viewpoint of an airplane that is passing over Earth’s surface, the surface is seen to rotate slowly below. But, looking from the surface at the airplane, the surface seems stationary, and the airplane appears to curve off course. The airplane does not ac tually deviate from a straight path, but it appears to do so because we are standing on Earth’s rotating surface beneath the airplane. Be cause of this apparent deflection, the airplane must make constant corrections in flight path to main tain its “straight” heading relative to a rotating Earth (see Figure 6.7a and b).
A pilot leaves the North Pole and flies due south toward Quito, Ecuador. If Earth were not rotating, the aircraft would simply travel along a meridian of longitude and arrive at Quito. But Earth is rotat
High pressure
Isobar
(a) Pressure gradient, isobars, and wind strength.
Low pressure
Gradual pressure gradient
LIGHT WINDS
Steep pressure gradient
L
H 1024
Gradual pressure gradient
400 KILOMETRES
0 200
(b) Pressure gradient and wind strength portrayed on a weather map.
▲Figure 6.6 Effect of pressure gradient on wind speed.
winds would move in a straight line from areas of higher pressure to areas of lower pressure. But on our rotating planet, the Coriolis force deflects anything that flies or flows across Earth’s surface—wind, an airplane, or ocean currents—from a straight path. Because Earth rotates eastward, such objects appear to curve to the right in the Northern Hemisphere and to the left in the Southern Hemisphere. Because the speed of Earth rotation varies with latitude, the strength of this deflection varies, being weakest at the equator and strongest at the poles.
Note that we call Coriolis a force. This label is ap propriate because, as the physicist Sir Isaac Newton
ing eastward beneath the aircraft’s flight path. As the plane travels toward the equator, the speed of Earth’s ro tation increases from about 838 km·h−1 at 60° N to about 1675 km·h−1 at 0°. If the pilot does not allow for this in crease in rotational speed, the plane will reach the equa tor over the ocean along an apparently curved path, far to the west of the intended destination (Figure 6.7a). On the return flight northward, if the pilot does not make cor rections, the plane will end up to the east of the pole, in a righthand deflection.
This effect also occurs if the plane is flying in an east–west direction. During an eastward flight from Vancouver to Gander, in the same direction as Earth’s rotation, the centrifugal force pulling outward on the
1028 mb
1024 mb
1020 mb
1016 mb
1012 mb
1008 mb
1004 mb 1000 mb
1012 mb
Strong winds
1028
1004
1000
Light winds
1020 mb
1016
1008 mb