Page 140 - Algebra

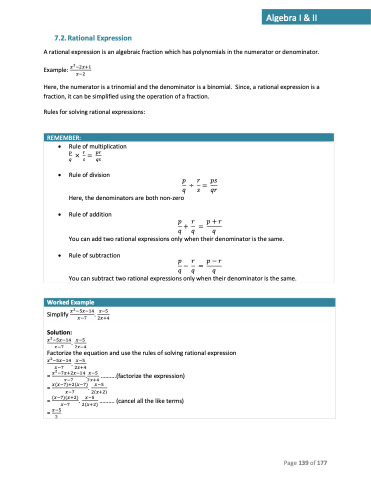
7.2. Rational Expression
A rational expression is an algebraic fraction which has polynomials in the numerator or denominator. Example: 𝑥2−2𝑥+1
𝑥−2
Here, the numerator is a trinomial and the denominator is a binomial. Since, a rational expression is a fraction, it can be simplified using the operation of a fraction.
Rules for solving rational expressions:
REMEMBER:
• Rule of multiplication 𝑝 × 𝑟 = 𝑝𝑟
𝑞 𝑠 𝑞𝑠
• Rule of division
Here, the denominators are both non-zero
• Rule of addition
You can add two rational expressions only when their denominator is the same.
• Rule of subtraction
You can subtract two rational expressions only when their denominator is the same.
𝑝 ÷ 𝑟 = 𝑝𝑠 𝑞 𝑠 𝑞𝑟
𝑝+𝑟=𝑝+𝑟 𝑞𝑞𝑞
𝑝𝑟𝑝−𝑟
−= 𝑞𝑞𝑞
Worked Example
𝑥2−5𝑥−14 𝑥−5 Simplify 𝑥−7 . 2𝑥+4
Solution:
𝑥2−5𝑥−14 𝑥−5
Factorize the equation and use the rules of solving rational expression
𝑥2−5𝑥−14 𝑥−5
= 𝑥−5 2
𝑥−7
.
2𝑥−4
𝑥−7
.
2𝑥+4
𝑥2−7𝑥+2𝑥−14 𝑥−5
= 𝑥−7 .2𝑥+4 ..........(factorize the expression)
𝑥(𝑥−7)+2(𝑥−7) 𝑥−5 = 𝑥−7 . 2(𝑥+2)
(𝑥−7)(𝑥+2) 𝑥−5
= 𝑥−7 . 2(𝑥+2) .......... (cancel all the like terms)
Page 139 of 177
Algebra I & II