Page 35 - Algebra

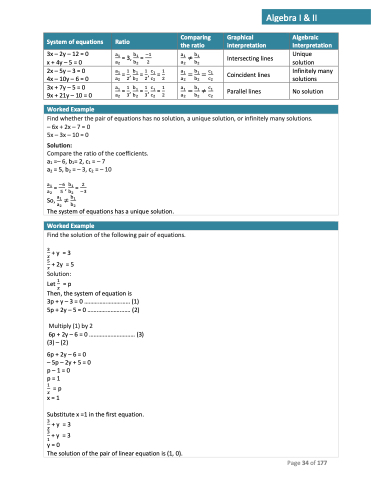
System of equations
Ratio
Comparing the ratio
Graphical interpretation
Algebraic Interpretation
3x – 2y – 12 = 0 x + 4y – 5 = 0
2x – 5y – 3 = 0 4x – 10y – 6 = 0 3x + 7y – 5 = 0 9x + 21y – 10 = 0
a1 b1 a2 = 3, b2
a1 = 1, b1 a2 2 b2
a1 = 1, b1 a2 3 b2
−1 a1 =2 a2
=1,c1 =1 a1 2c2 2 a2
=1,c1 =1 a1 3c2 2 a2
b1 ≠ b2
= b1 = c1 b2 c2
= b1 ≠ c1 b2 c2
Intersecting lines Coincident lines Parallel lines
Unique solution Infinitely many solutions
No solution
Worked Example
Find whether the pair of equations has no solution, a unique solution, or infinitely many solutions.
– 6x + 2x – 7 = 0
5x – 3x – 10 = 0
Solution:
Compare the ratio of the coefficients.
a1 =– 6, b1= 2, c1 = – 7
a2 = 5, b2 = – 3, c2 = – 10
a1 −6b1 2
=,=
a2 5 b2 −3
So,a1 ≠b1 a2 b2
The system of equations has a unique solution.
Worked Example
Find the solution of the following pair of equations.
3+y=3 𝑥
5 + 2y = 5 𝑥
Solution:
Let1 =p 𝑥
Then, the system of equation is 3p + y – 3 = 0 .............................. (1) 5p + 2y – 5 = 0 ............................ (2)
Multiply (1) by 2
6p + 2y – 6 = 0 .............................. (3) (3) – (2)
6p + 2y – 6 = 0 – 5p – 2y + 5 = 0 p–1=0
p =1
1=p 𝑥
x= 1
Substitute x =1 in the first equation.
3+y=3 𝑥
3+y=3 1
y=0
The solution of the pair of linear equation is (1, 0).
Page 34 of 177
Algebra I & II