Page 97 - Algebra

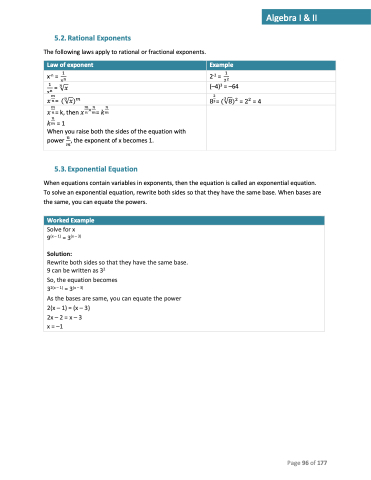
5.2. Rational Exponents
The following laws apply to rational or fractional exponents.
x-n = 1 xn
1 𝑛 xn = √𝑥
2-2 = 1 22
(–4)3 = –64
Law of exponent
Example
𝑚𝑛𝑚 2322 𝑥𝑛=(√𝑥) 83=(√8) =2 =4
5.3. Exponential Equation
When equations contain variables in exponents, then the equation is called an exponential equation. To solve an exponential equation, rewrite both sides so that they have the same base. When bases are the same, you can equate the powers.
𝑚 𝑚×𝑛𝑛
𝑥𝑛 = k, then 𝑥𝑛 𝑚= 𝑘𝑚 𝑛
𝑘𝑚 = 1
When you raise both the sides of the equation with
power 𝑛 , the exponent of x becomes 1. 𝑚
Worked Example
Solve for x
9(x–1) =3(x–3)
Solution:
Rewrite both sides so that they have the same base.
9 can be written as 32
So, the equation becomes
32(x–1) =3(x–3)
As the bases are same, you can equate the power
2(x – 1) = (x – 3)
2x – 2 = x – 3
x = –1
Page 96 of 177
Algebra I & II