Page 102 - IM_FL_Geometry_Print Sample

Student Task Statement
Choose a decimal between 0 and 3 to the tenths place (for example, 1.5). Sketch a large circle with a central angle whose radian measure is equal to the decimal you chose. Be prepared to explain your reasoning.
Student Response
Answers vary. Any sketch should have an arc length that is their chosen number multiplied by the radius.
Activity Synthesis
Ask one or two students to share their responses and explain how they were able to sketch their circle. Display a variety of responses between 0 and 3 to illustrate that this range of radians spans almost half a circle, regardless of the size of the circles.
3.2 Arcs and Angles
15 minutes
The purpose of this activity is for students to develop the understanding that the measure of a central angle ranges between 0 radians and radians, regardless of the size of the circle. Just as students have become accustomed to degree measures between 0 and 360, they will build familiarity with radian measures between 0 and .
Look for di erent strategies for answering question 5. One strategy would be to notice that the radian measure is the full measure of an entire circle, , being divided by 3 and calculate of the
circumference to nd the arc length. Another strategy would be to multiply the radius by the radian measure to nd the arc length.
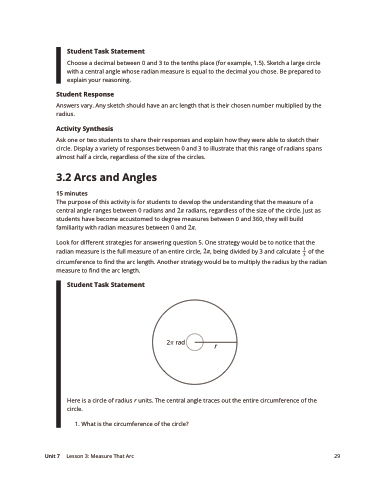
Student Task Statement
Here is a circle of radius units. The central angle traces out the entire circumference of the circle.
1. What is the circumference of the circle?
Unit 7 Lesson 3: Measure That Arc 29