Page 144 - IM_FL_Geometry_Print Sample

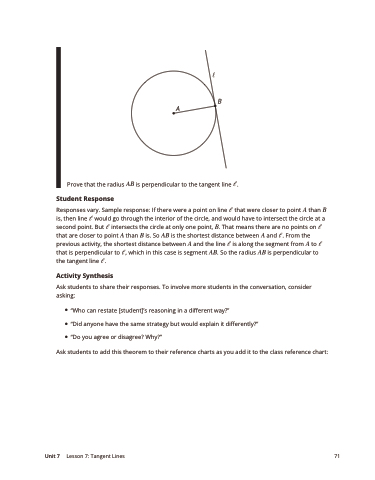
Prove that the radius is perpendicular to the tangent line .
Student Response
Responses vary. Sample response: If there were a point on line that were closer to point than is, then line would go through the interior of the circle, and would have to intersect the circle at a second point. But intersects the circle at only one point, . That means there are no points on that are closer to point than is. So is the shortest distance between and . From the previous activity, the shortest distance between and the line is along the segment from to that is perpendicular to , which in this case is segment . So the radius is perpendicular to the tangent line .
Activity Synthesis
Ask students to share their responses. To involve more students in the conversation, consider asking:
• “Who can restate [student]’s reasoning in a di erent way?”
• “Did anyone have the same strategy but would explain it di erently?” • “Do you agree or disagree? Why?”
Ask students to add this theorem to their reference charts as you add it to the class reference chart:
Unit 7 Lesson 7: Tangent Lines 71