Page 155 - IM_FL_Geometry_Print Sample

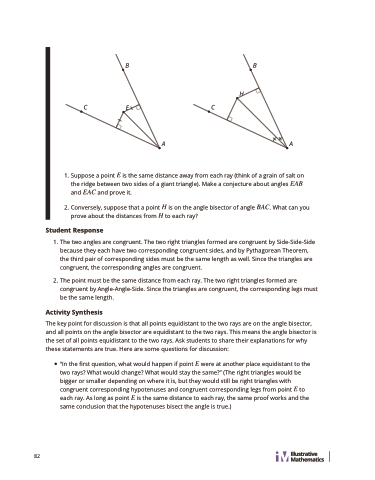
1. Suppose a point is the same distance away from each ray (think of a grain of salt on the ridge between two sides of a giant triangle). Make a conjecture about angles
and and prove it.
2. Conversely, suppose that a point is on the angle bisector of angle . What can you prove about the distances from to each ray?
Student Response
1. The two angles are congruent. The two right triangles formed are congruent by Side-Side-Side because they each have two corresponding congruent sides, and by Pythagorean Theorem, the third pair of corresponding sides must be the same length as well. Since the triangles are congruent, the corresponding angles are congruent.
2. The point must be the same distance from each ray. The two right triangles formed are congruent by Angle-Angle-Side. Since the triangles are congruent, the corresponding legs must be the same length.
Activity Synthesis
The key point for discussion is that all points equidistant to the two rays are on the angle bisector, and all points on the angle bisector are equidistant to the two rays. This means the angle bisector is the set of all points equidistant to the two rays. Ask students to share their explanations for why these statements are true. Here are some questions for discussion:
• “In the rst question, what would happen if point were at another place equidistant to the two rays? What would change? What would stay the same?” (The right triangles would be bigger or smaller depending on where it is, but they would still be right triangles with congruent corresponding hypotenuses and congruent corresponding legs from point to each ray. As long as point is the same distance to each ray, the same proof works and the same conclusion that the hypotenuses bisect the angle is true.)
82
Teacher Guide