Page 166 - IM_FL_Geometry_Print Sample

Student Task Statement
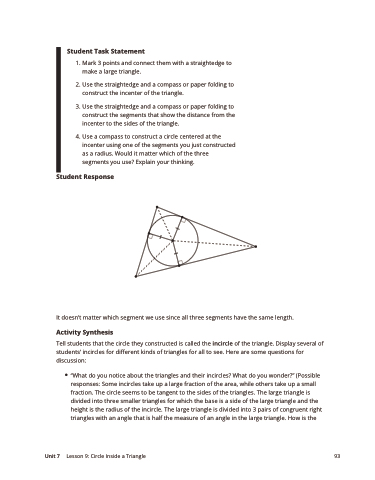
1. Mark 3 points and connect them with a straightedge to make a large triangle.
2. Use the straightedge and a compass or paper folding to construct the incenter of the triangle.
3. Use the straightedge and a compass or paper folding to construct the segments that show the distance from the incenter to the sides of the triangle.
4. Use a compass to construct a circle centered at the incenter using one of the segments you just constructed as a radius. Would it matter which of the three segments you use? Explain your thinking.
Student Response
Unit 7
Lesson 9: Circle Inside a Triangle 93
It doesn’t matter which segment we use since all three segments have the same length.
Activity Synthesis
Tell students that the circle they constructed is called the incircle of the triangle. Display several of students’ incircles for di erent kinds of triangles for all to see. Here are some questions for discussion:
• “What do you notice about the triangles and their incircles? What do you wonder?” (Possible responses: Some incircles take up a large fraction of the area, while others take up a small fraction. The circle seems to be tangent to the sides of the triangles. The large triangle is divided into three smaller triangles for which the base is a side of the large triangle and the height is the radius of the incircle. The large triangle is divided into 3 pairs of congruent right triangles with an angle that is half the measure of an angle in the large triangle. How is the