Page 180 - IM_FL_Geometry_Print Sample

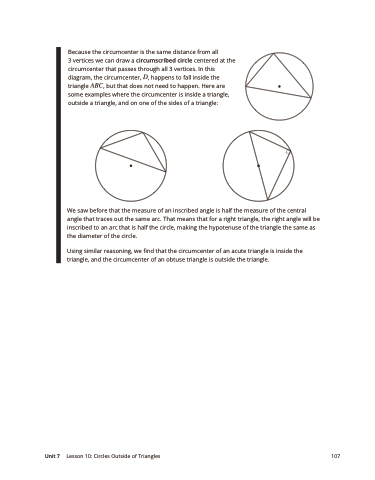
Because the circumcenter is the same distance from all
3 vertices we can draw a circumscribed circle centered at the circumcenter that passes through all 3 vertices. In this diagram, the circumcenter, , happens to fall inside the triangle , but that does not need to happen. Here are some examples where the circumcenter is inside a triangle, outside a triangle, and on one of the sides of a triangle:
Unit 7
Lesson 10: Circles Outside of Triangles 107
We saw before that the measure of an inscribed angle is half the measure of the central angle that traces out the same arc. That means that for a right triangle, the right angle will be inscribed to an arc that is half the circle, making the hypotenuse of the triangle the same as the diameter of the circle.
Using similar reasoning, we nd that the circumcenter of an acute triangle is inside the triangle, and the circumcenter of an obtuse triangle is outside the triangle.