Page 191 - IM_FL_Geometry_Print Sample

Lesson 11 Practice Problems
Problem Statement
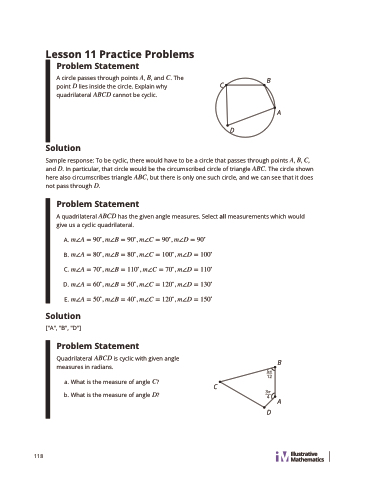
A circle passes through points , , and . The point lies inside the circle. Explain why quadrilateral cannot be cyclic.
Solution
Sample response: To be cyclic, there would have to be a circle that passes through points , , , and . In particular, that circle would be the circumscribed circle of triangle . The circle shown here also circumscribes triangle , but there is only one such circle, and we can see that it does not pass through .
Problem Statement
A quadrilateral has the given angle measures. Select all measurements which would give us a cyclic quadrilateral.
A. B. C. D. E.
Solution
["A", "B", "D"]
Problem Statement
Quadrilateral is cyclic with given angle measures in radians.
a. What is the measure of angle ? b. What is the measure of angle ?
118
Teacher Guide