Page 91 - IM_FL_Geometry_Print Sample

for this lesson because students will use the fact that all circles are similar to de ne radian measure as a constant of proportionality.
Student Task Statement
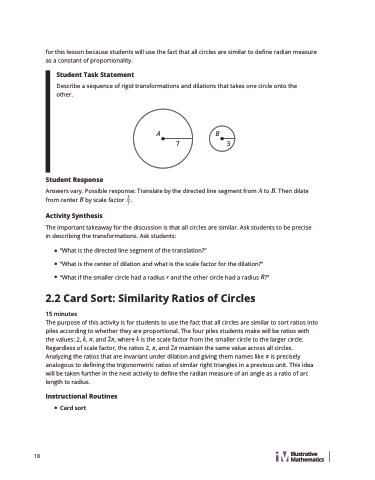
Describe a sequence of rigid transformations and dilations that takes one circle onto the other.
Student Response
Answers vary. Possible response: Translate by the directed line segment from to . Then dilate from center by scale factor .
Activity Synthesis
The important takeaway for the discussion is that all circles are similar. Ask students to be precise in describing the transformations. Ask students:
• “What is the directed line segment of the translation?”
• “What is the center of dilation and what is the scale factor for the dilation?” • “What if the smaller circle had a radius and the other circle had a radius ?”
2.2 Card Sort: Similarity Ratios of Circles
15 minutes
The purpose of this activity is for students to use the fact that all circles are similar to sort ratios into piles according to whether they are proportional. The four piles students make will be ratios with the values: 2, , , and , where is the scale factor from the smaller circle to the larger circle. Regardless of scale factor, the ratios 2, , and maintain the same value across all circles. Analyzing the ratios that are invariant under dilation and giving them names like is precisely analogous to de ning the trigonometric ratios of similar right triangles in a previous unit. This idea will be taken further in the next activity to de ne the radian measure of an angle as a ratio of arc length to radius.
Instructional Routines
• Card sort
18
Teacher Guide