Page 153 - IM_Algebra2_FL Print Sample.pdf
P. 153
Student Lesson Summary
Situations can lead to the recursive de nition or closed form de nition for a sequence.
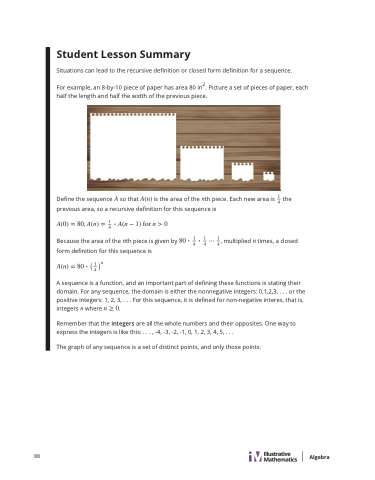
For example, an 8-by-10 piece of paper has area 80 in2. Picture a set of pieces of paper, each half the length and half the width of the previous piece.
De ne the sequence so that is the area of the th piece. Each new area is the previous area, so a recursive de nition for this sequence is
Because the area of the th piece is given by , multiplied times, a closed form de nition for this sequence is
A sequence is a function, and an important part of de ning these functions is stating their domain. For any sequence, the domain is either the nonnegative integers: 0,1,2,3, . . . or the positive integers: 1, 2, 3, . . . For this sequence, it is de ned for non-negative interes, that is, integers where .
Remember that the integers are all the whole numbers and their opposites. One way to express the integers is like this: . . . , -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, . . .
The graph of any sequence is a set of distinct points, and only those points:
88
Teacher Guide
Algebra