Page 159 - MATHEMATICS COURSE FOR SECONDARY SCHOOLS BOOK 2

The scale ‘1 cm 􏰀 3 km’ means that a distance of 1 centimetre on the map represents a distance of 3 kilometres on the ground.
So the length of the drawing = ––1– × 1400 = 7. 200
That is, the length of the drawing is 7 cm.
The width of the drawing : 1000 = 1 : 200
The scale: 1 cm 􏰀 3 km may be given as the Scales
A scale of 1 cm to 2 m means t1hat every
map ratio 1 : 300000 or as the representative
So the width of the drawing = ––– × 1000 = 5. 200
1 fraction ––––––.
2 metres in the room is represented by
300 000
1 centimetre on the drawing.
That is, the width of the drawing is 5 cm.
1 cm : 3 km = 1 cm : 300 000 cm
= 1 : 300 000 Please
This means that: supply this
distance on the marptw: odrikstance of the ground = 1 : 300 000
This is a ratio.
A ratio is a comparison between two quantities expressed in the same unit.
Scale: 1 cm 􏰀 3 km Drawing to scale
The scale ‘1 cm 􏰀 3 km’ means that a distance
of 1 centimetre on the map represents a
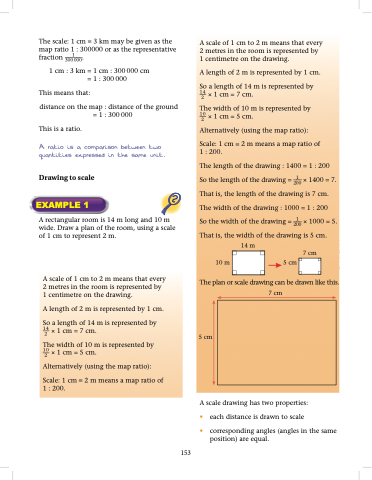
14 m
Please supply
distance of 3 kilometres on the ground.
EXAMPLE 1
Example
The scale: 1 cm 􏰀 3 km may be given as the A rectangular room is 14 m long and 10 m
A length of 2 m is represented by 1 cm.
7 cm
So a length of 14 m is represented by this
14 10 m 5 cm artwork –– × 1 cm = 7 cm.
2
ThTeheplwanidotrhscoafle10drmawisnrgecparnesbeendteradwbnylike this.
–1–0 × 1 cm = 5 cm. 2
7 cm
Alternatively (using the map ratio):
Scale: 1 cm 􏰀 2 m means a map ratio of 1 : 200.
So the length of the drawing = ––1– × 1400 = 7. 200
That is, the length of the drawing is 7 cm. The width of the drawing : 1000 = 1 : 200 So the width of the drawing = ––1– × 1000 = 5.
5 cm
The length of the drawing : 1400 = 1 : 200
A scale of 1 cm to 2 m means that every distance on the map : distance of the ground
2 metres in the room is represented by = 1 : 300 000
1 centimetre on the drawing.
This is a ratio.
A length of 2 m is represented by 1 cm.
A ratio is a comparison between two
So a length of 14 m is represented by
q–1u–4antities expressed in the same unit. 2 × 1 cm = 7 cm.
The width of 10 m is represented by
D–1–0r×aw1icnmgt=o5scamle. 2
Alternatively (using the map ratio):
1 : 200.
A rectangular room is 14 m long and 10 m
Example
Scale: 1 cm 􏰀 2 m means a map ratio of
distance a
d.
as the ntative
So the length of the drawing = ––– × 1400 = 7. 200
􏰁 􏰁
Please
each distance is drawn to scale
corresponding angles (angles in the same position) are equal.
383
map ratio 1 : 300000 or as the representative
200 A scale drawing has two properties:
wide. Draw a plan of the room, using a scale 1
fraction ––––––. 300 000
That is, the width of the drawing is 5 cm. each distance is drawn to scale
correspo
of 1 cm to represent 2 m.
􏰁 􏰁
Please supply this artwork
383
1 cm : 3 km = 1 cm : 300 000 cm = 1 : 300 000
This means that:
14 m
The length of the drawing : 1400 = 1 : 200 wide. Draw a plan of the room, using a scale
5 cm
A scale drawing has two properties:
of 1 cm to represent 2 m. 1
That is, the length of the drawing is 7 cm. The width of the drawing : 1000 = 1 : 200
1 153 So the width of the drawing = ––– × 1000 = 5.
200
That is, the width of the drawing is 5 cm.
10 m
es (angles in the same
5 cm
position
nding angl ) are equal.
The plan or scale drawing can be drawn like this. 7 cm
7 cm
s e
14 m