Page 76 - MATHEMATICS COURSE FOR SECONDARY SCHOOLS BOOK 2

E
E
338
The centre of a circle
The point exactly at the middle of a circle is called its centre.
thicknesses of the tape. Remove the tape from around the object and use a metric ruler to measure its length.
length represent?
To make the set squares rest squarely on
the ruler, you may need to start your measurement from a calibration that is not zero. You must take this into account when you measure the diameter.
The centre of a circle is the point that is
equidistance from all points on the circumference.
The radius of a circle
Any straight line drawn from the centre of a circle to its circumference is a radius of the circle. The plural of radius is radii.
A radius of a circle is the line from the centre to a point on the circumference.
The radius of a circle is denoted by the letter r. How many radii can a circle have?
The diameter of a circle
The longest straight line that can be drawn from one point on the circumference to another is called a diameter of the circle. A diameter always passes through the centre of the circle.
A diameter of a circle is a straight line passing through the centre of the circle and meeting the circumference in two places.
The diameter of a circle is denoted by the letter d. How many diameters can a circle have?
The centre of a circle is usually labelled O.
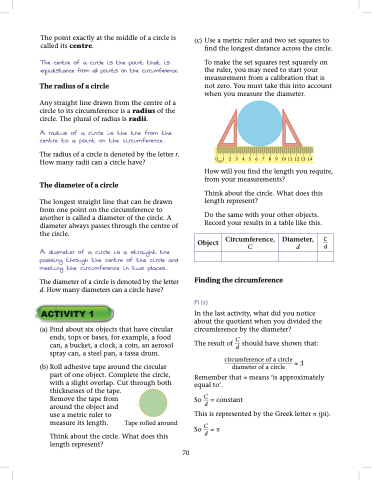
(c) Use a metric ruler and two set squares to fiTnhdinthkealboonugtetsht edicsitracnlec.eWachraotssdothees tchiricsle.
Tape rolled around
The va
􏰀 is an termin
For ex
1
–= 0 5 2
–1 = 0.3 3
To sim usuall
The fo circum
Since Then
The fo in ter
Making
Show t
Express radius
Each d radii i
So the d=
􏰂d=
Since Then So C =
The fo in ter
Making
Show t
e ial
How will you find the length you require, from your measurements?
Think about the circle. What does this length represent?
Do the same with your other objects. Record your results in a table like this.
Object Circumference, Diameter, Cd Cd
Finding the circumference
Pi (􏰀)
In the last activity, what did you notice about the quotient when you divided the circumference by the diameter?
The result of C should have shown that: d
circumference of a circle 􏰁 3 diameter of a circle
Remember that 􏰁 means ‘is approximately equal to’.
So Cd = constant
This is represented by the Greek letter 􏰀 (pi).
SoCd=􏰀
. Mark he line.
de a B.
ter of f the
th of a
0 1 2 3 4 5 6 7 8 9 1011121314
cm
ACTIVITY 1
Activity 10
(a) Find about six objects that have circular ends, tops or bases, for example, a food can, a bucket, a clock, a coin, an aerosol spray can, a steel pan, a tassa drum.
(b) Roll adhesive tape around the circular part of one object. Complete the circle, with a slight overlap. Cut through both thicknesses of the tape.
rcle is
t is ference.
Remove the tape from around the object and use a metric ruler to measure its length.
Tape rolled around
Think about the circle. What does this length represent?
70
N
S
N
a
3
p y
r
B
h t
m
a A
e o
C
r m
C Sd
g sn
y
r
2
C C
r m
i
m