Page 37 - modul trigonometri_Neat

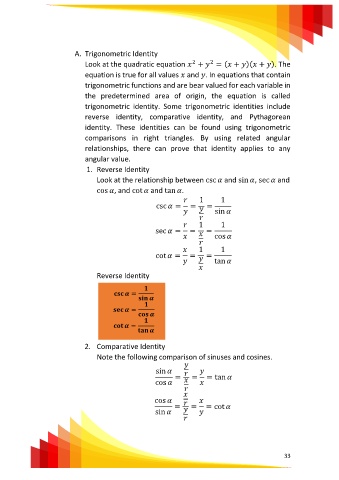
A. Trigonometric Identity
2
2
Look at the quadratic equation + = ( + )( + ). The
equation is true for all values and . In equations that contain
trigonometric functions and are bear valued for each variable in
the predetermined area of origin, the equation is called
trigonometric identity. Some trigonometric identities include

reverse identity, comparative identity, and Pythagorean
identity. These identities can be found using trigonometric

comparisons in right triangles. By using related angular
relationships, there can prove that identity applies to any
angular value.
1. Reverse Identity

Look at the relationship between csc and sin , sec and
cos , and cot and tan .
1 1
csc = = =
sin

1 1
sec = = =
cos

1 1
cot = = =
tan

Reverse Identity

=

=

=

2. Comparative Identity

Note the following comparison of sinuses and cosines.

sin
= = = tan
cos

cos
= = = cot
sin

33