Page 123 - classs 6 a_Neat

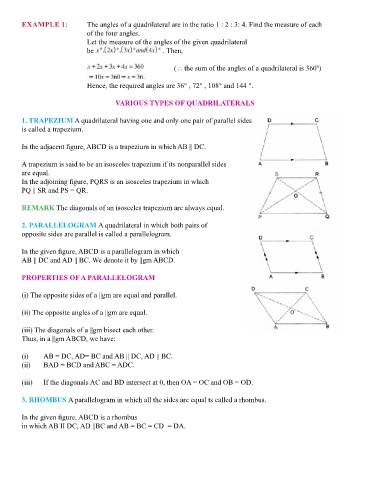
CONVEX AND CONCAVE QUADRILATERALS EXAMPLE 1: The angles of a quadrilateral are in the ratio 1 : 2 : 3: 4. Find the measure of each
of the four angles.
CONVEX QUADRILATERAL A quadrilateral in which the measure of each angle is less than 180° Let the measure of the angles of the given quadrilateral
is called a convex quadrtlateral. be . Then,

( the sum of the angles of a quadrilateral is 360 )
0

Hence, the required angles are 36° , 72° , 108° and 144 °.

VARIOUS TYPES OF QUADRILATERALS

1. TRAPEZIUM A quadrilateral having one and only one pair of parallel sides
is called a trapezium.
CONCAVE QUADRILATERAL A quadrilateral in which the measure of one of the angles is more
than 180 is called a concave quadrilateral. In the adjacent figure, ABCD is a trapezium in which AB || DC.

A trapezium is said to be an isosceles trapezium if its nonparallel sides
are equal.
In the adjoining figure, PQRS is an isosceles trapezium in which
PQ || SR and PS = QR.

REMARK The diagonals of an isosceles trapezium are always equal.

2. PARALLELOGRAM A quadrilateral in which both pairs of
In the above figure, PQRS is a concave quadrilateral in.which LS> 180° . In this chapter, by a quadrilateral opposite sides are parallel is called a parallelogram.
we would mean a convex quadrilateral.
In the given figure, ABCD is a parallelogram in which
INTERIOR AND EXTERIOR OF A QUADRILATERAL AB || DC and AD || BC. We denote it by ||gm ABCD.

Consider a quadrilateral ABCD. It divides the whole plane into three parts. PROPERTIES OF A PARALLELOGRAM
(i) The part of the plane lying inside the boundary ABCD is called the
interior of the quadrilateral ABCD. Each point of this part is called (i) The opposite sides of a ||gm are equal and parallel.
an interior point of the quadrilateral. In the given figure,
the points P, Q, R are the interior points of the quadrilateral ABCD. (ii) The opposite angles of a ||gm are equal.

(ii) The part of the plane lying outside the boundary ABCD is called (iii) The diagonals of a ||gm bisect each other.
the exterior of the quadrilateral ABCD. Each point of this part is Thus, in a ||gm ABCD, we have:
called an exterior point of the quadrilateral. A E
(i) AB = DC, AD= BC and AB || DC, AD || BC.
In the given figure, the points Land Mare the exterior points of the quadrilateral ABCD. (ii) BAD = BCD and ABC = ADC.
(iii) The boundary ABCD.
(iii) If the diagonals AC and BD intersect at 0, then OA = OC and OB = OD.
Clearly, the point E lies on the quadrilateral ABCD.
3. RHOMBUS A parallelogram in which all the sides are equal ts called a rhombus.
QUADRILATERAL REGION The interior of the quadrilateral ABCD together with its boundary is
called the quadrilateral region ABCD. In the given figure, ABCD is a rhombus
in which AB II DC, AD ||BC and AB = BC = CD = DA.
ANGLE SUM PROPERTY OF A QUADRILATERAL The sum of the angles of a quadrtlateral is 360° .