Page 133 - classs 6 a_Neat

In the above figure, ∆ LMN is a right triangle, as ∆LMN = 90 •
0
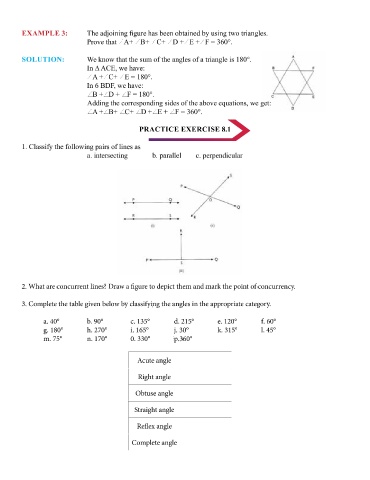
EXAMPLE 3: The adjoining figure has been obtained by using two triangles.
(iii) OBTUSE TRIANGLE A triangle one of whose angles measures more than 90 is called an Prove that A+ B+ C+ D + E + F = 360°.
0
obtuse-angled triangle or simply an obtuse triangle.
SOLUTION: We know that the sum of the angles of a triangle is 180°.
In ∆ ACE, we have:
A + C+ E = 180°.
In 6 BDF, we have:
B + D + F = 180°.
Adding the corresponding sides of the above equations, we get:
A + B+ C+ D + E + F = 360°.

In the above figure, ∆PQR is obtuse. So, ∆PQR is an obtuse triangle. PRACTICE EXERCISE 8.1

SOME IMPORTANT RESULTS 1. Classify the following pairs of lines as
a. intersecting b. parallel c. perpendicular
RESULT 1. Each angle of an equilateral triangle measures 60°.

RESULT 2. The angles opposite to equal sides of an isosceles triangle are equal.

RESULT 3. A scalene triangle has no two angles equal.

ANGLE SUM PROPERTY OF A TRIANGLE

The sum of the angles of a triangle is 180°, or 2 right angles.
As a consequence of the above result, we can say that

(i) a triangle cannot have more than one right angle,
(ii) a triangle cannot have more than one obtuse angle,
(iii) in a right triangle, the sum of the two acute angles is 90 •
0
2. What are concurrent lines? Draw a figure to depict them and mark the point of concurrency.
ILLUSTRATIVE EXAMPLES
3. Complete the table given below by classifying the angles in the appropriate category.
EXAMPLE 1: Find the angles of a triangle which are in the ratio 2 : 3 : 4.
a. 40° b. 90° c. 135° d. 215° e. 120° f. 60°
SOLUTION: Let the measures of the given angles be g. 180° h. 270° i. 165° j. 30° k. 315° l. 45°
Then m. 75° n. 170° 0. 330° p.360°
Hence, the measures of the angles of the given triangle are 40°, 60° and 80°
Acute angle
EXAMPLE 2: In a ∆ABC, if 2 A = 3 B = 6 C then calculate A, B and C.

Right angle
SOLUTION: Let
Obtuse angle

Then Straight angle

Reflex angle

Complete angle