Page 37 - classs 6 a_Neat

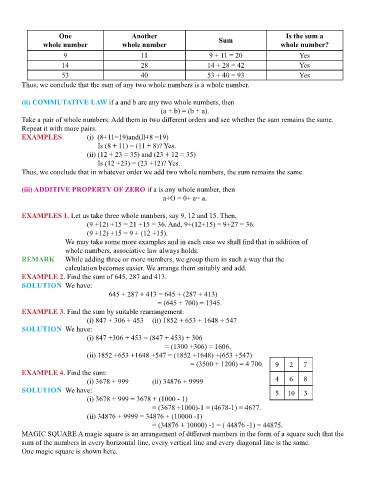
Is the sum a
Another
One
3 WHOLE NUMBERS whole number whole number Sum whole number?
9 11 9 + 11 = 20 Yes
14 28 14 + 28 = 42 Yes
53 40 53 + 40 = 93 Yes
NATURAL NUMBERS We are already familiar with the counting numbers 1, 2, 3, 4, 5, 6, etc. Thus, we conclude that the sum of any two whole numbers is a whole number.
Counting numbers are called natural numbers.

(ii) COMMUTATIVE LAW if a and b are any two whole numbers, then
WHOLE NUMBERS All natural numbers together with ‘O’ are called whole numbers. (a + b) = (b + a).

Take a pair of whole numbers. Add them in two different orders and see whether the sum remains the same.
Thus 0, 1, 2, 3, 4, 5, 6, 7, 8, 10, 11, 12, ... are whole numbers. Repeat it with more pairs.
Clearly, every natural number is a whole number but 0 is a whole number which is not a natural number. EXAMPLES (i) (8+11=19)and(ll+8 =19)

Is (8 + 11) = (11 + 8)? Yes.
SUCCESSOR OF A WHOLE NUMBER if we add 1 to a whole number, we get the next whole (ii) (12 + 23 = 35) and (23 + 12 = 35)
number, called its successor. Is (12 +23) = (23 +12)? Yes.
Thus, we conclude that in whatever order we add two whole numbers, the sum remains the same.
Thus, the successor of 0 is 1, the successor of 1 is 2, the successor of 12 is 13, and so on. Every whole number
has its successor. (iii) ADDITIVE PROPERTY OF ZERO if a is any whole number, then

a+O = 0+ a= a.
PREDECESSOR OF A WHOLE NUMBER One less than a given whole number (other than 0), is
called its predecessor.
EXAMPLES 1. Let us take three whole numbers, say 9, 12 and 15. Then,
(9 +12) +15 = 21 +15 = 36. And, 9+(12+15) = 9+27 = 36.
Thus, the predecessor of 1 is 0, the predecessor of 2 is 1, the predecessor of 10 is 9, and so on. The whole (9 +12) +15 = 9 + (12 +15).
number 0 does not have its predecessor. We may take some more examples and in each case we shall find that in addition of
Every whole number other than 0 has its predecessor. whole numbers, associative law always holds.
Example: Write the successor and predecessor of REMARK While adding three or more numbers, we group them in such a way that the
(i) 1000 (ii) 1005399 (iii) 999999 calculation becomes easier. We arrange them suitably and add.
EXAMPLE 2. Find the sum of 645, 287 and 413.
Solution: (i) The successor of l000 = (1000 + 1) = 1001. SOLUTION We have:
The predecessor of l000 = (1000 -1) = 999. 645 + 287 + 413 = 645 + (287 + 413)
(ii) The successor of 1005399 = (1005399 + 1) = 1005400. = (645 + 700) = 1345.
The predecessor ofl005399 = (1005399 -1) = 1005398. EXAMPLE 3. Find the sum by suitable rearrangement:
(iii) The successor of 999999 = (999999 + 1) = 1000000. (i) 847 + 306 + 453 (ii) 1852 + 653 + 1648 + 547
The predecessor of 999999 = (999999 - 1) = 999998. SOLUTION We have:

(i) 847 +306 + 453 = (847 + 453) + 306
OPERATIONS ON WHOLE NUMBERS = (1300 +306) = 1606.
(ii) 1852 +653 +1648 +547 = (1852 +1648) +(653 +547)
We are already familiar with the four basic operations of addition, subtraction, multiplication and division on = (3500 + 1200) = 4 700. 9 2 7
whole numbers. Now, we shall study the properties of these operations on whole numbers. EXAMPLE 4. Find the sum:
(i) 3678 + 999 (ii) 34876 + 9999 4 6 8
PROPERTIES OF ADDITION SOLUTION We have: 5 10 3
(i) 3678 + 999 = 3678 + (1000 - 1)
(i) CLOSURE PROPERTY if a and b are any two whole numbers, then (a+ b) is also a whole number. = (3678 +1000)-1 = (4678-1) = 4677.
Let us take some pairs of whole numbers and add them. Check whether the sum is a whole number. (ii) 34876 + 9999 = 34876 + (10000 -1)

= (34876 + 10000) -1 = ( 44876 -1) = 44875.
MAGIC SQUARE A magic square is an arrangement of dtfferent numbers in the form of a square such that the
sum of the numbers in every horizontal line, every vertical line and every diagonal line is the same.
One magic square is shown here.