Page 44 - classs 6 a_Neat

Let us observe some other interesting patterns in natural numbers.
0 is not a counting number. It is not a natural number. a. 1 × 8 + 1 = 9
When we add Oto any natural number, we get a new set of numbers called whole numbers. This set of whole 12 × 8 + -2 = 98
numbers is denoted by Wand is written as W = {O, 1, 2, 3, 4 ... } 123 -× 8 + -3 = 987

Thus, natural numbers or and whole numbers or 1,234 × 8 + 1 = 9,876
N = {1,2,3,4,5 ... } 12,345 × 8 + 5 = 98,765
W = {0, 1, 2, 3, 4, 5 ... }. 1,23,456 × 8 + 6 = 9,87,654
The smallest natural number is 1. The smallest whole number is 0. and so on.

b. 1 × 9 + 2 =11
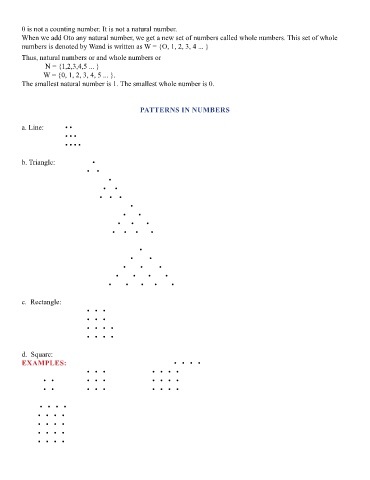
PATTERNS IN NUMBERS 12 × 9 + 3 =111
1 23 × 9 + 4 = 1,111
a. Line: • • 1,234 × 9 + 5 = 11,111
• • • 12,345 × 9 + 6 = 1,11,111
• • • • 1,23,456 × 9 + 7 = 11,11,111
and so on.
b. Triangle: •
• • c. 9 X 9 + 7 = 88
• 98 × 9 + 6 = 888
• • 987 × 9 + 5 = 8,888
• • • 9,876 × 9 + 4 = 88,888
• 98,765 × 9 + 3 = 8,88,888
• • 9,87,654 × 9 + 2 = 88,88,888
• • • and so on.
• • • •
d. (11) = 121
2
• (111) = 12,321
2
• • (1,111) = 12,34,321
2
• • • (11,111) = 12,34,54,321
2
• • • • and so on.
• • • • •
e. 1 = 1 × 2 1+ 2 + 3 + 4 = 4 ×5
c. Rectangle: 2 2
• • •
• • • 1 + 2 = 2 × 3 1 + 2 + 3 + 4 + 5 = 5 × 6
• • • • 2 2
• • • • 1 + 2 + 3 = 3 × 4 and so on.
2
d. Square: PRACTICE EXERCISE 3.1
EXAMPLES: • • • • 1. Which property do the following represent?
• • • • • • • a. 17 + 18 = 18 + 17
• • • • • • • • • b. (20 + 22) + 24 = 20 + (22 + 24)
• • • • • • • • • c. 25 + 0 = 25 = 0 + 25

d. (15 × 17) × 16 = 15 × (17× 16)
• • • • e. 25 × 22 = 22 × 25
• • • • f. 41 × 1 = 41 = 1 × 41
• • • • g. 9 × (8 + 7) = 9 × 8 + 9 × 7
• • • • h. 5 × 20 = 100 = 20 × 5
• • • • i. 100 + 101 = 201 = 101 + 100
j. 16 + (8 × 7) = 16 + 8× 16 + 7