Page 9 - Nature Of Space And Time

a reasonable equation of state. It may not however be satis ed locally by the quantum
mechanical expectation value of the energy momentum tensor. This will be relevant in my
second and third lectures.
Suppose the weak energy condition holds, and that the null geodesics from a point p
begin to converge again and that has the positive value 0. Then the Newman Penrose

equation would imply that the convergence would become in nite at a point q within an
a ne parameter distance 1 if the null geodesic can be extended that far.
0

1
If = 0 at v = v 0 then ≥ . Thus there is a conjugate point
−1 +v 0 −v
before v = v 0 + −1 .

+
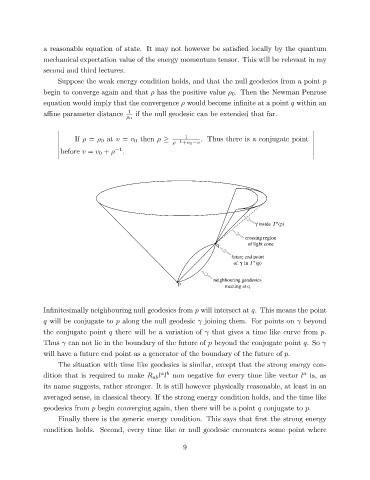
g inside (p)
I
crossing region
q of light cone

future end point
+
g
I
of in (p)
neighbouring geodesics
p
meeting at q

In nitesimally neighbouring null geodesics from p will intersect at q. This means the point

q will be conjugate to p along the null geodesic
joining them. For points on
beyond
the conjugate point q there will be a variation of
that gives a time like curve from p.
Thus
can not lie in the boundary of the future of p beyond the conjugate point q.So
will have a future end point as a generator of the boundary of the future of p.

The situation with time like geodesics is similar, except that the strong energy con-
a b
a
dition that is required to make R abl l non negative for every time like vector l is, as
its name suggests, rather stronger. It is still however physically reasonable, at least in an
averaged sense, in classical theory. If the strong energy condition holds, and the time like

geodesics from p begin converging again, then there will be a point q conjugate to p.
Finally there is the generic energy condition. This says that rst the strong energy
condition holds. Second, every time like or null geodesic encounters some point where

9