Page 158 - The Miracle of the Honeybee

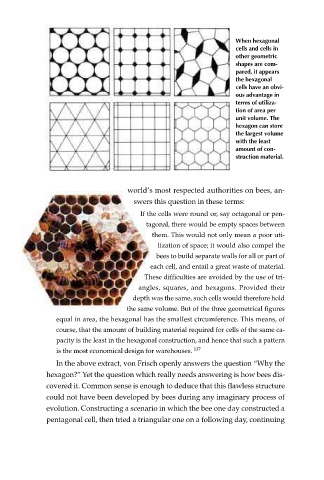
When hexagonal
cells and cells in
other geometric
shapes are com-
pared, it appears
the hexagonal
cells have an obvi-
ous advantage in
terms of utiliza-
tion of area per
unit volume. The
hexagon can store
the largest volume
with the least
amount of con-
struction material.

world’s most respected authorities on bees, an-
swers this question in these terms:

If the cells were round or, say octagonal or pen-
tagonal, there would be empty spaces between
them. This would not only mean a poor uti-
lization of space; it would also compel the
bees to build separate walls for all or part of
each cell, and entail a great waste of material.
These difficulties are avoided by the use of tri-
angles, squares, and hexagons. Provided their
depth was the same, such cells would therefore hold
the same volume. But of the three geometrical figures
equal in area, the hexagonal has the smallest circumference. This means, of
course, that the amount of building material required for cells of the same ca-
pacity is the least in the hexagonal construction, and hence that such a pattern
is the most economical design for warehouses. 137
In the above extract, von Frisch openly answers the question “Why the
hexagon?” Yet the question which really needs answering is how bees dis-
covered it. Common sense is enough to deduce that this flawless structure
could not have been developed by bees during any imaginary process of
evolution. Constructing a scenario in which the bee one day constructed a
pentagonal cell, then tried a triangular one on a following day, continuing