Page 209 - Geosystems An Introduction to Physical Geography 4th Canadian Edition

aQuantitativeSOlUTiOn Wind Regimes
The wind regime for a location can be described by summariz- ing records of wind speed and direction for a given time period. Mean wind speed (u) can be calculated as a simple average:
u = 1 / n an u i i = 1
where u is wind speed, n is the total number of observations, and © is the symbol for summation. It is possible to calculate a value for the mean wind direction—known as a wind resultant—by us- ing vector analysis and some basic trigonometric functions.
A wind rose is a circular plot that shows the relative distribu- tion of wind direction (from which the wind was blowing), with each petal on the rose portraying data for a range of com-
pass directions. The compass is usually divided into 8, 16, or
36 segments, corresponding to ranges of 45o, 22.5o, or 10o, respectively. It is possible to describe the annual wind regime for a place, or the regime for a specific part of the year, such as a particular season or month. When displaying a wind rose, it is important to include the period over which data were collected, and the part of the year that is represented.
Annual wind roses for two locations in Ontario—Windsor and Kapuskasing—for the 30-year period from 1976–2005 are pre- sented in Figure AQS 6.1. By plotting both sets of data with the same scales, a visual comparison of the long-term wind regimes can be made. Kapuskasing is located 800 km directly north of Windsor, and comparing the wind roses shows that it has a differ- ent wind regime than Windsor. The data shown represent a sum- mary of almost 263 000 hourly measurements for each location that were collected by the Meteorological Service of Canada1 and used to create the Canadian Weather Energy and Engineering (CWEEDS) data set (with data for most stations available up to 2005). In addition to the directional information, the frequency
of wind speeds within each direction category is displayed with coloured segments on the petals.
1Wind data source: Canadian Weather energy and engineering Datasets (CWeeDS), ftp://ftp.tor.ec.gc.ca/Pub/Engineering_ Climate_Dataset/Canadian_Weather_Energy_Engineering_ Dataset_CWEEDS_2005/ZIPPED%20FILES/ENGLISH/ONTARIO .zip.
xai i=1
n
V = -1/n sinu
Vy = -1/nan cosui i=1
Vx and Vy are the east–west and north–south components of the resultant and is u wind direction. The wind resultant direction (u) is calculated by:
u = arctan(Vx/Vy) + C
The value of C depends on the result of arctan (Vx / Vy ). If the result is > 180o, then C = +180o; if the result is > 180o, then C = −180o. This last step is necessary to produce an answer that describes the mean direction from which the wind blows.
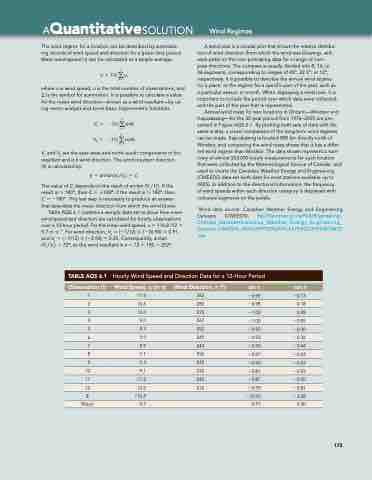
Table AQS 6.1 contains a sample data set to show how mean wind speed and direction are calculated for hourly observations over a 12-hour period. For the mean wind speed, u = 116.8 /12 = 9.7 m · s−1. For wind direction, Vx = (−1/12) × (−10.90) = 0.91, and Vy = (−1/12) × (−3.58) = 0.30. Consequently, arctan
(Vx /Vy) = 72o, so the wind resultant is u = 72 + 180 = 252o.
TABLE AQS 6.1
Hourly Wind Speed and Direction Data for a 12-Hour Period
Observation (i)
Wind Speed, ui (m s)
Wind Direction, ui (°)
sin ui
cos ui
1 11.6 3 12.4 5 9.3 7 8.9 9 5.3
11 11.2  116.8
262
274
252
244
245
240
−0.99 −0.13 −1.00 0.08 −0.95 −0.30 −0.90 −0.44 −0.90 −0.43 −0.87 −0.50
−10.90 −3.58
2
10.6
280
−0.98
0.18
4
9.0
267
−1.00
−0.05
6
9.9
249
−0.93
−0.36
8
7.1
256
−0.97
−0.23
10
9.1
234
−0.81
−0.59
12
12.5
216
−0.59
−0.81
Mean
9.7
0.91
0.30
173