Page 17 - Math 21 Module_Chapter One

9

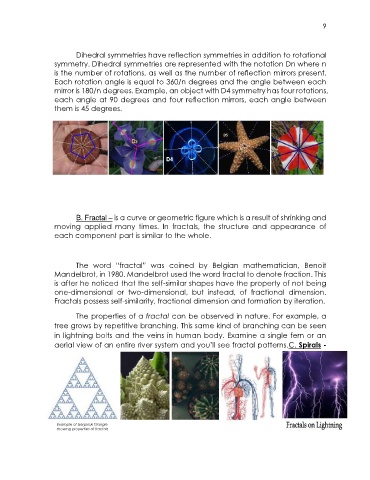
Dihedral symmetries have reflection symmetries in addition to rotational
symmetry. Dihedral symmetries are represented with the notation Dn where n
is the number of rotations, as well as the number of reflection mirrors present.
Each rotation angle is equal to 360/n degrees and the angle between each
mirror is 180/n degrees. Example, an object with D4 symmetry has four rotations,
each angle at 90 degrees and four reflection mirrors, each angle between
them is 45 degrees.

B. Fractal – is a curve or geometric figure which is a result of shrinking and
moving applied many times. In fractals, the structure and appearance of
each component part is similar to the whole.

The word “fractal” was coined by Belgian mathematician, Benoit
Mandelbrot, in 1980. Mandelbrot used the word fractal to denote fraction. This
is after he noticed that the self-similar shapes have the property of not being
one-dimensional or two-dimensional, but instead, of fractional dimension.
Fractals possess self-similarity, fractional dimension and formation by iteration.

The properties of a fractal can be observed in nature. For example, a
tree grows by repetitive branching. This same kind of branching can be seen
in lightning bolts and the veins in human body. Examine a single fern or an
aerial view of an entire river system and you’ll see fractal patterns.C. Spirals -

Example of Sierpinski Triangle
showing properties of fractals