Page 133 - IM_FL_Geometry_Print Sample

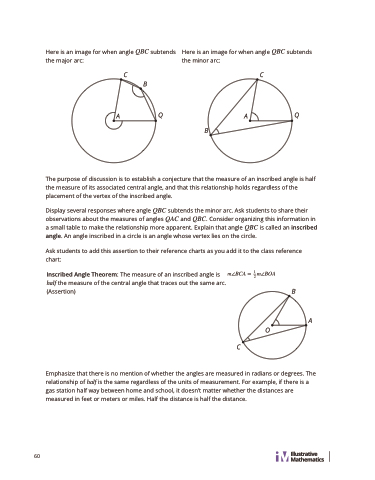
Here is an image for when angle subtends Here is an image for when angle subtends
the major arc:
the minor arc:
The purpose of discussion is to establish a conjecture that the measure of an inscribed angle is half the measure of its associated central angle, and that this relationship holds regardless of the placement of the vertex of the inscribed angle.
Display several responses where angle subtends the minor arc. Ask students to share their observations about the measures of angles and . Consider organizing this information in a small table to make the relationship more apparent. Explain that angle is called an inscribed angle. An angle inscribed in a circle is an angle whose vertex lies on the circle.
Ask students to add this assertion to their reference charts as you add it to the class reference chart:
Inscribed Angle Theorem: The measure of an inscribed angle is half the measure of the central angle that traces out the same arc. (Assertion)
Emphasize that there is no mention of whether the angles are measured in radians or degrees. The relationship of half is the same regardless of the units of measurement. For example, if there is a gas station half way between home and school, it doesn’t matter whether the distances are measured in feet or meters or miles. Half the distance is half the distance.
60
Teacher Guide