Page 135 - IM_FL_Geometry_Print Sample

Activity Synthesis
Ask students to share their explanations. Record their explanations for all to see and ask if your recording accurately captures their arguments.
Lesson Synthesis
In this lesson, students conjectured that the measure of an inscribed angle is half the measure of the central angle that traces out the same arc. This would be a good opportunity to connect back to the right triangle whose hypotenuse is the diameter of a circle. Display an image of a circle with a diameter drawn and its center marked for all to see. Mark a point on the circle that is not one of the endpoints of the diameter. Draw chords that connect this point to the endpoints of the diameter. Ask students, “Where is an inscribed angle in this picture? Where is the central angle that traces out the same arc?” For ease of communication, ask students to label points and angles as they explain their reasoning. Ask, “according to the inscribed angle theorem, what does the measure of the inscribed angle have to be?”
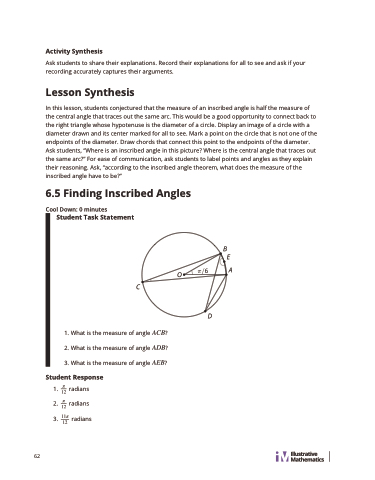
6.5 Finding Inscribed Angles
Cool Down: 0 minutes
Student Task Statement
1. What is the measure of angle
2. What is the measure of angle ?
3. What is the measure of angle
Student Response
1. radians 2. radians 3. radians
? ?
62
Teacher Guide