Page 158 - IM_FL_Geometry_Print Sample

Activity Synthesis
The key idea to discuss is that there are points on segment that are closer to side than the other two sides, and there are points closer to sides and , and somewhere between those extremes, there is a point that is the same distance away from all three sides. Ask students to share their responses to the nal two questions. Ask students to clarify their explanations using precise language, if necessary. Tell students that the name for this special point that is the same distance from each side of a triangle is incenter.
Lesson Synthesis
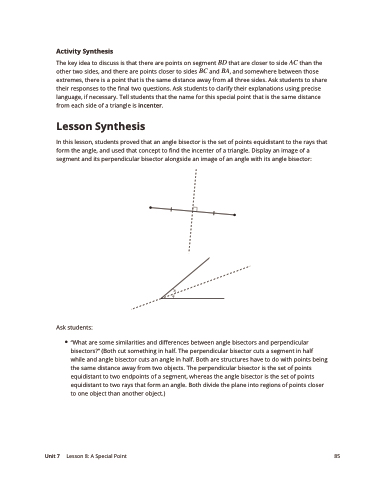
In this lesson, students proved that an angle bisector is the set of points equidistant to the rays that form the angle, and used that concept to nd the incenter of a triangle. Display an image of a segment and its perpendicular bisector alongside an image of an angle with its angle bisector:
Unit 7
Lesson 8: A Special Point 85
Ask students:
• “What are some similarities and di erences between angle bisectors and perpendicular bisectors?” (Both cut something in half. The perpendicular bisector cuts a segment in half while and angle bisector cuts an angle in half. Both are structures have to do with points being the same distance away from two objects. The perpendicular bisector is the set of points equidistant to two endpoints of a segment, whereas the angle bisector is the set of points equidistant to two rays that form an angle. Both divide the plane into regions of points closer to one object than another object.)