Page 96 - IM_FL_Geometry_Print Sample

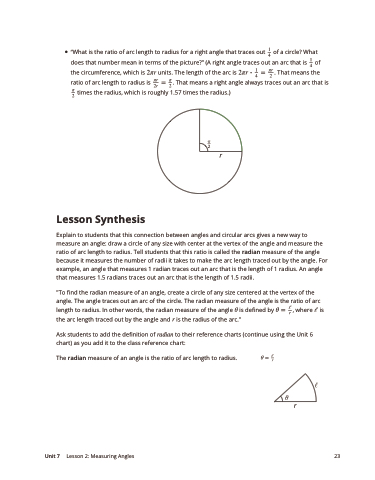
• “What is the ratio of arc length to radius for a right angle that traces out of a circle? What does that number mean in terms of the picture?” (A right angle traces out an arc that is of the circumference, which is units. The length of the arc is . That means the ratio of arc length to radius is . That means a right angle always traces out an arc that is
times the radius, which is roughly 1.57 times the radius.)
Lesson Synthesis
Explain to students that this connection between angles and circular arcs gives a new way to measure an angle: draw a circle of any size with center at the vertex of the angle and measure the ratio of arc length to radius. Tell students that this ratio is called the radian measure of the angle because it measures the number of radii it takes to make the arc length traced out by the angle. For example, an angle that measures 1 radian traces out an arc that is the length of 1 radius. An angle that measures 1.5 radians traces out an arc that is the length of 1.5 radii.
"To nd the radian measure of an angle, create a circle of any size centered at the vertex of the angle. The angle traces out an arc of the circle. The radian measure of the angle is the ratio of arc length to radius. In other words, the radian measure of the angle is de ned by , where is the arc length traced out by the angle and is the radius of the arc."
Ask students to add the de nition of radian to their reference charts (continue using the Unit 6 chart) as you add it to the class reference chart:
The radian measure of an angle is the ratio of arc length to radius.
Unit 7 Lesson 2: Measuring Angles
23