Page 97 - IM_FL_Geometry_Print Sample

2.4 Reasoning with Radians
Cool Down: 5 minutes
Students who think Jada’s angle is larger probably think the radian measure depends somehow on the size of the circle, and are not calculating the ratio of arc length to radius.
Student Task Statement
Mai measures an angle that makes an arc length of centimeters at a radius of centimeters. Jada measures an angle that makes an arc length of feet at a radius of feet.
1. What is the measure of their angles in radians?
2. Whose angle is larger? Explain your reasoning.
Student Response
1. Mai’s angle measures . Jada’s angle measures . 2. Mai’s is larger since of is more than of .
Student Lesson Summary
Up until this point, we have been thinking about measuring angles by what fraction of a circle the angle covers. Each degree covers of a full circle. For example, an angle measuring
degrees covers of a full circle. There is no geometric signi cance to the number 360—it is
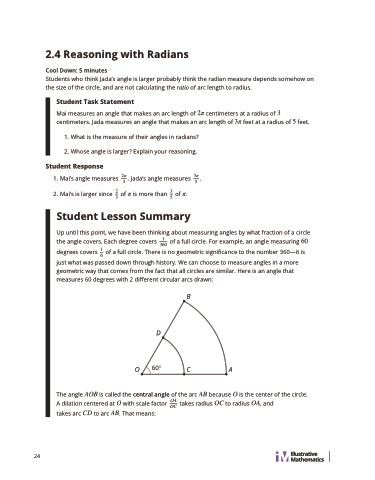
just what was passed down through history. We can choose to measure angles in a more geometric way that comes from the fact that all circles are similar. Here is an angle that measures 60 degrees with 2 di erent circular arcs drawn:
The angle is called the central angle of the arc because is the center of the circle. A dilation centered at with scale factor takes radius to radius , and
takes arc to arc . That means:
24
Teacher Guide