Page 10 - Three New Tables for an Astrology of Three Dimensions

It should be obvious that any imputed “influence” of the sun could
not be the same in both relative solar positions, but the flat horoscope
hides the discrepancy.
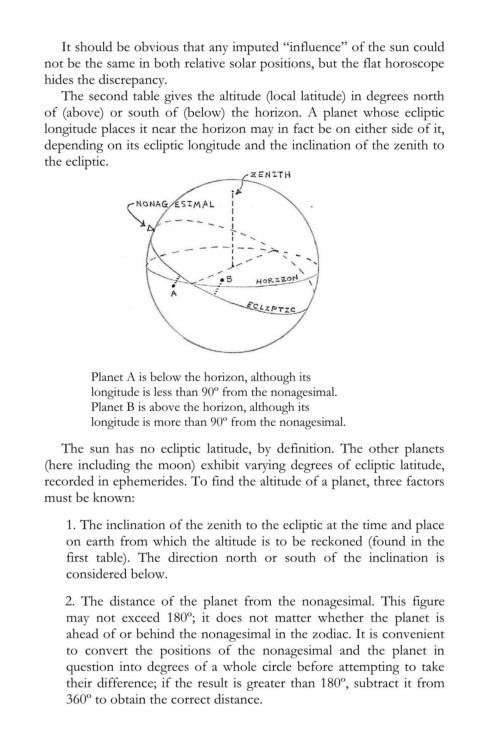
The second table gives the altitude (local latitude) in degrees north
of (above) or south of (below) the horizon. A planet whose ecliptic
longitude places it near the horizon may in fact be on either side of it,
depending on its ecliptic longitude and the inclination of the zenith to
the ecliptic.

Planet A is below the horizon, although its
longitude is less than 90º from the nonagesimal.
Planet B is above the horizon, although its
longitude is more than 90º from the nonagesimal.

The sun has no ecliptic latitude, by definition. The other planets
(here including the moon) exhibit varying degrees of ecliptic latitude,
recorded in ephemerides. To find the altitude of a planet, three factors
must be known:

1. The inclination of the zenith to the ecliptic at the time and place
on earth from which the altitude is to be reckoned (found in the
first table). The direction north or south of the inclination is
considered below.

2. The distance of the planet from the nonagesimal. This figure
may not exceed 180º; it does not matter whether the planet is
ahead of or behind the nonagesimal in the zodiac. It is convenient
to convert the positions of the nonagesimal and the planet in
question into degrees of a whole circle before attempting to take
their difference; if the result is greater than 180º, subtract it from
360º to obtain the correct distance.