Page 16 - PowerPoint 演示文稿

6 Ophthalmic Lenses

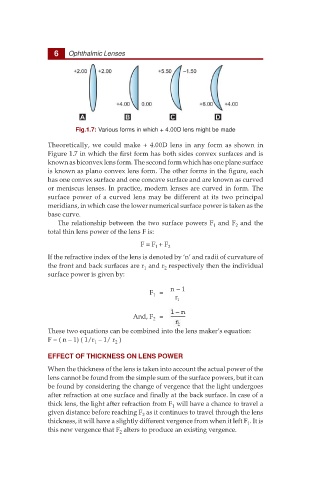
Fig.1.7: Various forms in which + 4.00D lens might be made
Theoretically, we could make + 4.00D lens in any form as shown in
Figure 1.7 in which the first form has both sides convex surfaces and is
known as biconvex lens form. The second form which has one plane surface
is known as plano convex lens form. The other forms in the figure, each
has one convex surface and one concave surface and are known as curved
or meniscus lenses. In practice, modern lenses are curved in form. The
surface power of a curved lens may be different at its two principal
meridians, in which case the lower numerical surface power is taken as the
base curve.
The relationship between the two surface powers F and F and the
2
1
total thin lens power of the lens F is:
F = F + F 2
1
If the refractive index of the lens is denoted by ‘n’ and radii of curvature of
the front and back surfaces are r and r respectively then the individual
2
1
surface power is given by:
F = n– 1
1
r
1
And, F = r
2
These two equations can be combined into the lens maker’s equation:
F = ( n – 1) ( 1/r – 1/ r )
1
2
EFFECT OF THICKNESS ON LENS POWER
When the thickness of the lens is taken into account the actual power of the
lens cannot be found from the simple sum of the surface powers, but it can
be found by considering the change of vergence that the light undergoes
after refraction at one surface and finally at the back surface. In case of a
thick lens, the light after refraction from F will have a chance to travel a
1
given distance before reaching F as it continues to travel through the lens
2
thickness, it will have a slightly different vergence from when it left F . It is
1
this new vergence that F alters to produce an existing vergence.
2