Page 90 - merged.pdf

•70 The 100 Greatest Business Ideas of All Time

If someone borrows £100 now, they will owe interest of £10 by the end of one year
so the total outstanding will be £110.

During the second year, interest will be charged on the total amount outstand-
ing of £110 i.e. interest of £11. The total outstanding would be £121.

During the third year, interest will be charged on the total amount outstanding
of £121 i.e. interest of £12.10. The total outstanding would then be £133.10.

We can see therefore that for every £100 borrowed, £133.10 must be repaid.
Therefore, solving the equation:

£A × 1.331 = £10,000

will tell us how much can be borrowed now, i.e. about £7,510.
This technique can of course be generalised to deal with any rate of interest and

any time period.
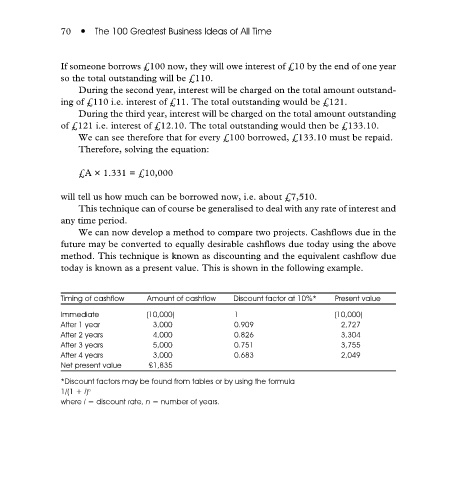
We can now develop a method to compare two projects. Cashflows due in the

future may be converted to equally desirable cashflows due today using the above
method. This technique is known as discounting and the equivalent cashflow due
today is known as a present value. This is shown in the following example.

Timing of cashflow Amount of cashflow Discount factor at 10%* Present value

Immediate (10,000) 1 (10,000)
After 1 year 3,000 0.909 2,727
After 2 years 4,000 0.826 3,304
After 3 years 5,000 0.751 3,755
After 4 years 3,000 0.683 2,049
Net present value
£1,835

*Discount factors may be found from tables or by using the formula
1/(1 + i)n
where i = discount rate, n = number of years.