Page 44 - Ramanujan Yatra

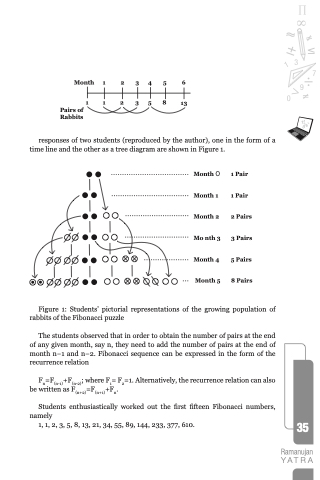
Month 1 2 3 4 5 6
Pairs of 1 1 2 3 5 8 13 Rabbits
responses of two students (reproduced by the author), one in the form of a time line and the other as a tree diagram are shown in Figure 1.
Month O Month 1 Month 2 Mo nth 3 Month 4
Month 5
1 Pair 1 Pair 2 Pairs 3 Pairs 5 Pairs 8 Pairs
Figure 1: Students’ pictorial representations of the growing population of rabbits of the Fibonacci puzzle
The students observed that in order to obtain the number of pairs at the end of any given month, say n, they need to add the number of pairs at the end of month n−1 and n−2. Fibonacci sequence can be expressed in the form of the recurrence relation
Fn=F(n-1)+F(n-2); where F1= F2=1. Alternatively, the recurrence relation can also be written as F(n+2)=F(n+1)+Fn.
Students enthusiastically worked out the first fifteen Fibonacci numbers, namely
1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610.
35
Ramanujan
YATRA