Page 53 - Ramanujan Yatra

Ramanujan
YATRA
44
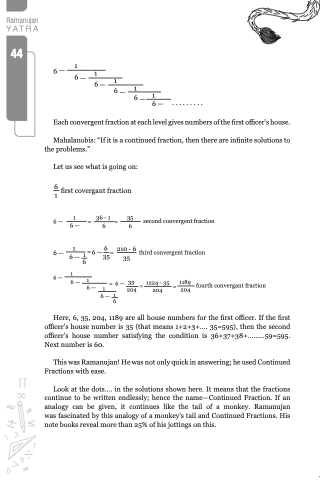
1 6—
Each convergent fraction at each level gives numbers of the first officer’s house.
Mahalanobis: “If it is a continued fraction, then there are infinite solutions to the problems.”
Let us see what is going on: 61 first covergant fraction
6—
1 6—
1 6—
11 6— 6—
6 —
6 —
6—
1 = 36 - 1 = 35 second convergent fraction 6— 6 6
1 1 = 6 — 6 = 210 - 6 third convergent fraction 6 — 6 35 35
. . . . .....
11
6 — 6 — 1 = 6 — 35 = 1224 - 35 = 1189 fourth convergant fraction
6 — 61 204 204 204
Here, 6, 35, 204, 1189 are all house numbers for the first officer. If the first officer’s house number is 35 (that means 1+2+3+.... 35=595), then the second officer’s house number satisfying the condition is 36+37+38+.........59=595. Next number is 60.
This was Ramanujan! He was not only quick in answering; he used Continued Fractions with ease.
Look at the dots.... in the solutions shown here. It means that the fractions continue to be written endlessly; hence the name—Continued Fraction. If an analogy can be given, it continues like the tail of a monkey. Ramanujan was fascinated by this analogy of a monkey’s tail and Continued Fractions. His note books reveal more than 25% of his jottings on this.