Page 20 - 091math-wb-w3-(sets)

WORKBOOK

2
Example 8: If A = { x : x  , x } and B = { x : x  4 , x }, then find A(n  ) B and
2
( n A ) B .

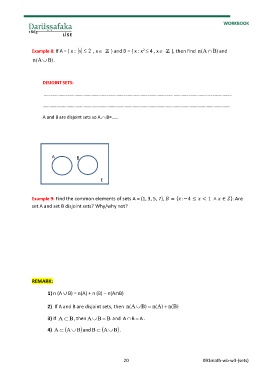
DISJOINT SETS:

…………………………………………………………………………………………………………………………………………………

…………………………………………………………………………………………………………………………………………………

A and B are disjoint sets so A B=……

A B

E

Example 9: Find the common elements of sets A = {1, 3, 5, 7}, = {: −4 ≤ < 1 ∧ ∈ }. Are
set A and set B disjoint sets? Why/why not?

REMARK:

1) n (A ∪ B) = n(A) + n (B) – n(A∩B)

2) If A and B are disjoint sets, then (n A ) B  ( n A )  ( n ) B

3) If A  B, then A B  B and A  B A .

4) A  A  B and B  A  B .

20 091math-wb-w3-(sets)