Page 225 - J. C. Turner "History and Science of Knots"

216 History and Science of Knots

can also be used to check the calculations; the sum of the coefficients in both
polynomials is equal to the number of regions in the diagram, which equals
(n + 2) by Euler's formula.
Listing's Complexions-Symbol has several serious defects. First, it is not
defined for the Unknot, when projected into a diagram having no crossings.
Nor is it defined for non-alternating knots, all of whose diagrams must have
at least one amphitypical region; examples of these occur first among knots
of eight crossings. The Prussian Heinrich Weith aus Homburg von der Hohe,
following up Listing's work, noted this in 1876 [93, pp. 15-16], and gave a
diagram of a non-alternating knot to prove the point.
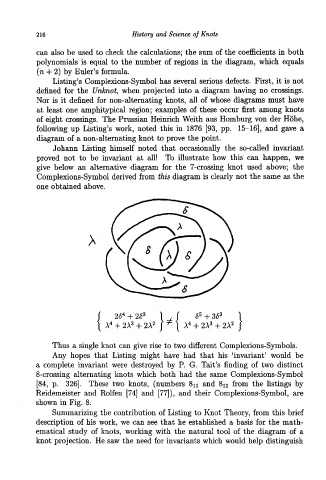
Johann Listing himself noted that occasionally the so-called invariant
proved not to be invariant at all! To illustrate how this can happen, we
give below an alternative diagram for the 7-crossing knot used above; the
Complexions-Symbol derived from this diagram is clearly not the same as the
one obtained above.

264 + 263 101 65 + 363
A4
+2A3+ 2A2 A4+2A3 +2A2

Thus a single knot can give rise to two different Complexions-Symbols.
Any hopes that Listing might have had that his `invariant' would be
a complete invariant were destroyed by P. G. Tait's finding of two distinct
8-crossing alternating knots which both had the same Complexions-Symbol
[84, p. 326]. These two knots, (numbers 81, and 812 from the listings by
Reidemeister and Rolfen [74] and [77]), and their Complexions-Symbol, are
shown in Fig. 8.
Summarizing the contribution of Listing to Knot Theory, from this brief
description of his work, we can see that he established a basis for the math-
ematical study of knots, working with the natural tool of the diagram of a
knot projection. He saw the need for invariants which would help distinguish