Page 256 - J. C. Turner "History and Science of Knots"

A History of Topological Knot Theory 247

P triggered off even more ambitious research, which came from roughly two
(interacting) directions. One of these was from physicists churning out link
invariants. The other was from the mathematical camp of knot-theorists,
trying hard to understand them.
It became obvious that via Markov's and Alexander's Theorem, one should
be able to relate algebraic interpretations for link invariants to the braid
groups. Algebraically, the link problem translates into domesticating a class
function on the Markov classes. However, a head-on attack on Markov equiv-
alence in B,,, is hopelessly difficult. Luckily, representation theory's richness
provides plenty of room for finding invariants. As the endpoints of braids
define a permutation in a natural way, the symmetric group S,, thus exists
as a quotient in Bn.. It is natural, then, to study how representations of S,b
and B. are related. It turns out that every irreducible representation of S,
transforms to a parametrised family of irreducible representations of B. In
fact, S,, transforms to an algebra H,,(q), the so-called Hecke algebra, when
q -> 1.

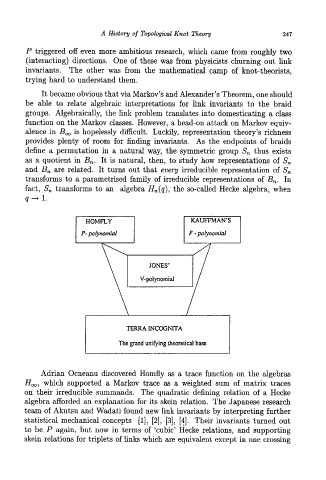
HOMFLY KAUFFMAN'S
P- polynomial F - polynomial

JONES'

V-polynomial

TERRA INCOGNITA

The grand unifying theoretical base

Adrian Ocneanu discovered Homfly as a trace function on the algebras
H.., which supported a Markov trace as a weighted sum of matrix traces
on their irreducible summands. The quadratic defining relation of a Hecke
algebra afforded an explanation for its skein relation. The Japanese research
team of Akutsu and Wadati found new link invariants by interpreting further
statistical mechanical concepts [1], [2], [3], [4]. Their invariants turned out
to be P again, but now in terms of `cubic' Hecke relations, and supporting
skein relations for triplets of links which are equivalent except in one crossing