Page 219 - J. C. Turner - History and Science of Knots

A History of Topological Knot Theory 209

on various lengths of string, connected in ordered, meaningful ways, would
encourage deeper mathematical thought. The Incas were aware that their
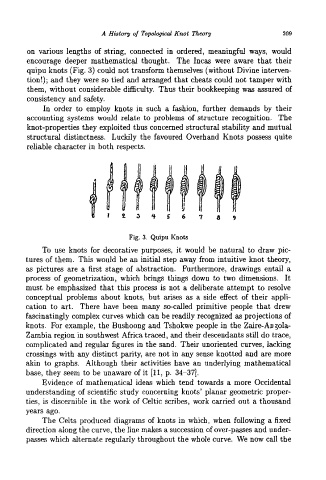
quipu knots (Fig. 3) could not transform themselves (without Divine interven-
tion!); and they were so tied and arranged that cheats could not tamper with
them, without considerable difficulty. Thus their bookkeeping was assured of
consistency and safety.
In order to employ knots in such a fashion, further demands by their
accounting systems would relate to problems of structure recognition. The
knot-properties they exploited thus concerned structural stability and mutual
structural distinctness. Luckily the favoured Overhand Knots possess quite
reliable character in both respects.

4

Fig. 3. Quipu Knots
To use knots for decorative purposes, it would be natural to draw pic-
tures of them. This would be an initial step away from intuitive knot theory,
as pictures are a first stage of abstraction. Furthermore, drawings entail a
process of geometrization, which brings things down to two dimensions. It
must be emphasized that this process is not a deliberate attempt to resolve
conceptual problems about knots, but arises as a side effect of their appli-
cation to art. There have been many so-called primitive people that drew
fascinatingly complex curves which can be readily recognized as projections of
knots. For example, the Bushoong and Tshokwe people in the Zaire-
Angola-Zambia region in southwest Africa traced, and their descendants still do trace,
complicated and regular figures in the sand. Their unoriented curves, lacking
crossings with any distinct parity, are not in any sense knotted and are more
akin to graphs. Although their activities have an underlying mathematical
base, they seem to be unaware of it [11, p. 34-37].
Evidence of mathematical ideas which tend towards a more Occidental
understanding of scientific study concerning knots' planar geometric proper-
ties, is discernible in the work of Celtic scribes, work carried out a thousand
years ago.
The Celts produced diagrams of knots in which, when following a fixed
direction along the curve, the line makes a succession of over-passes and under-
passes which alternate regularly throughout the whole curve. We now call the