Page 239 - J. C. Turner - History and Science of Knots
P. 239
A History of Topological Knot Theory 229
orientation. Let K C S3 be our given smooth knot. By thickening the knot's
actual curve to a knotted tube and removing this tube's interior from 3-space
we are left with X, the knot's exterior. By laying a `coordinate system' over
the tube around the knot, the exterior thus acquires more structure than the
complement. The exterior with the coordinate system is called the peripheral
system. Using additional information from the peripheral system Max Dehn
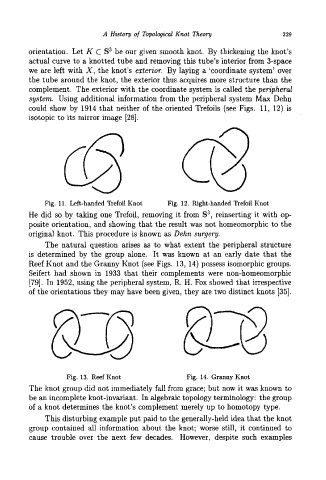
could show by 1914 that neither of the oriented Trefoils (see Figs. 11, 12) is
isotopic to its mirror image [28].
Fig. 11. Left-handed Trefoil Knot Fig. 12. Right-handed Trefoil Knot
He did so by taking one Trefoil, removing it from S3, reinserting it with op-
posite orientation, and showing that the result was not homeomorphic to the
original knot. This procedure is known as Dehn surgery.
The natural question arises as to what extent the peripheral structure
is determined by the group alone. It was known at an early date that the
Reef Knot and the Granny Knot (see Figs. 13, 14) possess isomorphic groups.
Seifert had shown in 1933 that their complements were non-homeomorphic
[79]. In 1952, using the peripheral system, R. H. Fox showed that irrespective
of the orientations they may have been given, they are two distinct knots [35].
Fig. 13. Reef Knot Fig. 14. Granny Knot
The knot group did not immediately fall from grace; but now it was known to
be an incomplete knot-invariant. In algebraic topology terminology: the group
of a knot determines the knot's complement merely up to homotopy type.
This disturbing example put paid to the generally-held idea that the knot
group contained all information about the knot; worse still, it continued to
cause trouble over the next few decades. However, despite such examples