Page 292 - J. C. Turner - History and Science of Knots

282 History and Science of Knots

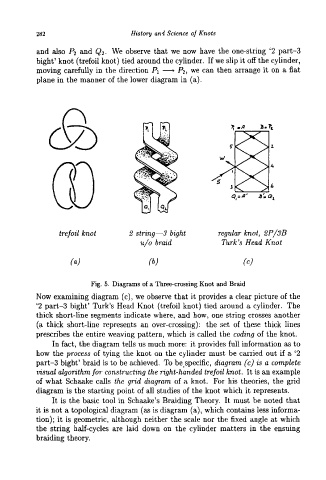
and also P2 and Q2. We observe that we now have the one-string `2 part-3
bight' knot (trefoil knot) tied around the cylinder. If we slip it off the cylinder,
moving carefully in the direction Pl -> P2, we can then arrange it on a flat
plane in the manner of the lower diagram in (a).

Co
CCZ

trefoil knot 2 string-3 bight regular knot, 2P/3B
u/o braid Turk's Head Knot

(c)

Fig. 5. Diagrams of a Three-crossing Knot and Braid
Now examining diagram (c), we observe that it provides a clear picture of the
`2 part-3 bight' Turk's Head Knot (trefoil knot) tied around a cylinder. The
thick short-line segments indicate where, and how, one string crosses another
(a thick short-line represents an over-crossing): the set of these thick lines
prescribes the entire weaving pattern, which is called the coding of the knot.
In fact, the diagram tells us much more: it provides full information as to
how the process of tying the knot on the cylinder must be carried out if a `2
part-3 bight' braid is to be achieved. To be,specific, diagram (c) is a complete
visual algorithm for constructing the right-handed trefoil knot. It is an example
of what Schaake calls the grid diagram of a knot. For his theories, the grid
diagram is the starting point of all studies of the knot which it represents.
It is the basic tool in Schaake's Braiding Theory. It must be noted that
it is not a topological diagram (as is diagram (a), which contains less informa-
tion); it is geometric, although neither the scale nor the fixed angle at which
the string half-cycles are laid down on the cylinder matters in the ensuing
braiding theory.