Page 298 - J. C. Turner - History and Science of Knots
P. 298
288 History and Science of Knots
formulae, algorithms and tables which solve many Wheelknot problems. He
has also discovered an evolution graph for Wheelknots which is `tree-like' but
contains cycles (i.e. it is not strictly a tree graph).
For Schaake's work to constitute a mathematical theory, it is necessary to
supply proofs of all the derived formulae and algorithms. In 1988, Schaake and
I began a program for writing out the proofs, in a Research Report Series, doing
this at the same time as producing the books and pamphlets on algorithms and
tables. The first two Reports, [20] and [21], written in 1988, covered the work
done on Regular Knots. At that point, several exciting mathematical projects
arose out of the evolution theory of Regular knots; in quick succession we wrote
books on number properties discovered from the Regular Knot Tree ([22], [24],
and [23]). The project for grinding out proofs of braiding algorithms fell into
abeyance; and it has not yet been restarted. We felt it more sensible to carry
on writing up pamphlets explaining the algorithms themselves. Schaake has
most of the proofs for his discoveries;. but it would take a further 2000 pages
of mathematical writing to present them all. He says `Let the mathematicians
who follow in my wake'-and he is sure that others will eventually do so-
`supply all the proofs. Keep them busy for a while!'
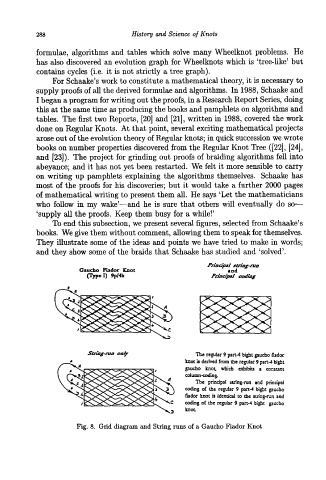
To end this subsection, we present several figures, selected from Schaake's
books. We give them without comment, allowing them to speak for themselves.
They illustrate some of the ideas and points we have tried to make in words;
and they show some of the braids that Schaake has studied and `solved'.
Priacip 1 :trio6 nra
Gaucho Piador Knot and
(type 1) 9p/4b Frmcip l 4vdio6
Stcio6 nro oo/y The regular 9 part-4 bight gaucho flador
knot is derived from the regular 9 part-4 bight
gaucho knot, which exhibits a constant
column-coding.
The principal string-run and principal
coding of the regular 9 part-4 bight gaucho
fiador knot is identical to the string-run and
coding of the regular 9 part-4 bight gaucho
knot.
Fig. 8. Grid diagram and String runs of a Gaucho Fiador Knot