Page 35 - J. C. Turner - History and Science of Knots

22 History and Science of Knots

Many of these variants in any one family have similar properties; they also
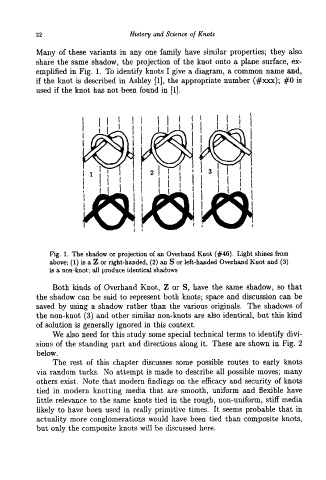
share the same shadow, the projection of the knot onto a plane surface, ex-
emplified in Fig. 1 . To identify knots I give a diagram , a common name and,
if the knot is described in Ashley [1], the appropriate number (#xxx); #0 is
used if the knot has not been found in [1].

' I I 2 (^ I I g i

i t ^( I I I ' I I ^ ^ i t

Fig. 1. The shadow or projection of an Overhand Knot (#46). Light shines from
above; (1) is a Z or right-handed, (2) an S or left-handed Overhand Knot and (3)
is a non-knot; all produce identical shadows

Both kinds of Overhand Knot, Z or S, have the same shadow, so that
the shadow can be said to represent both knots; space and discussion can be
saved by using a shadow rather than the various originals. The shadows of
the non-knot (3) and other similar non-knots are also identical, but this kind
of solution is generally ignored in this context.
We also need for this study some special technical terms to identify divi-
sions of the standing part and directions along it. These are shown in Fig. 2
below.
The rest of this chapter discusses some possible routes to early knots
via random tucks. No attempt is made to describe all possible moves; many
others exist. Note that modern findings on the efficacy and security of knots
tied in modern knotting media that are smooth, uniform and flexible have
little relevance to the same knots tied in the rough, non-uniform, stiff media
likely to have been used in really primitive times. It seems probable that in
actuality more conglomerations would have been tied than composite knots,
but only the composite knots will be discussed here.