Page 286 - MATHEMATICS COURSE FOR SECONDARY SCHOOLS BOOK 2

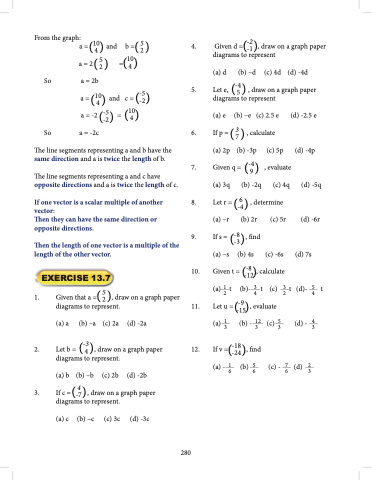
From the graph:
So
same direction and a is twice the length of b. The line segments representing a and c have
opposite directions and a is twice the length of c. If one vector is a scalar multiple of another
vector:
Then they can have the same direction or opposite directions.
Then the length of one vector is a multiple of the length of the other vector.
EXERCISE 13.7
1. Given that a =(25 ), draw on a graph paper diagrams to represent.
(a) a (b) –a (c) 2a (d) -2a
(-2) ()() -1
a = 10 and b = 5 4. Given d = , draw on a graph paper
a = 2b
5 , draw on a graph paper (-4)
42
diagrams to represent
a = 2 5 = 10 () ()
24
(a) d
(b) –d (c) 4d (d) -4d
(-5) 4()
5. Let e,
diagrams to represent
a = 10 and c = () -2
10 a = -2 (-5 ) = 4
(a) e
6. If p = ( 73 ) , calculate
(d) -2.5 e (d) -4p
-2
The line segments representing a and b have the
(b) –e
(c) 2.5 e
So
a = -2c
2. Let b = (-3), draw on a graph paper 12. If v = , find 4()
diagrams to represent.
-24
(a) - 16 (b) 56 (c) - 76 (d) 23
(a) b (b) –b (c) 2b (d) -2b
3. If c =(4 ), draw on a graph paper -7
diagrams to represent.
(a) c (b) –c (c) 3c (d) -3c
280
(a) 2p (b) -3p
7. Given q = 9 (-4)
(c) 5p
, evaluate
(c) 4q
(a) –r 9. If s =
(b) 2r
(c) 5r
(c) -6s
(a) 3q
(b) -2q
(d) -5q
(d) -6r (d) 7s
(d)- 54 t (d) - 4
8. Let r = ( 6 ) , determine -4
(a) –s
10. Given t =
(a) 12 t 11. Let u =
, calculate (-8)
(-8) -3
, find (b) 4s
-12
(b)- 34 t (c) 32 t
(-9) -15
-18
, evaluate (b) - 12 (c) 5
(a) 1 3333