Page 122 - Eric C. Fry - Knots and Ropework

Sen nits Whether they be flat, round, square or spira l, common,
English, French, Portuguese or Russian, sennits may be
broadly divided into three groups: those that can be made
w ith any number of strands; those that can only be made
with any odd number ; and those that require an even
number. The square sennit is an exception, fo r although
it requires an even number, it can be made only on eight,
twelve or sixteen strands or a multiple, eight being the
minimum.
A basic principle may be applied to the odd number group.
in that the strands are divided with one more on one side
than on the other, resulting in odd and even sides.
Thereafter the outside strands each time, starting with the
even side, are brought across to the centre and laid
inside the previous odd number ; thus the odd and even
sides alternate as the work proceeds.
Note
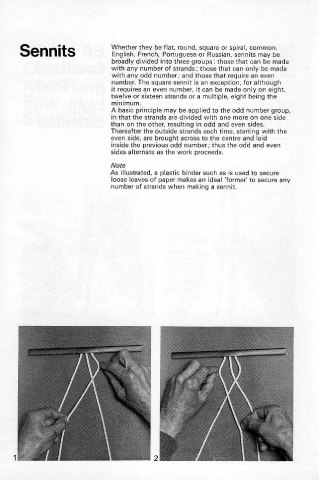
As illustrated, a plastic binder such as is used to secure
loose leaves of paper makes an ideal 'former" to secure any
number of strands when making a sennit.

1