Page 22 - MODULE 1 Q1MATH8

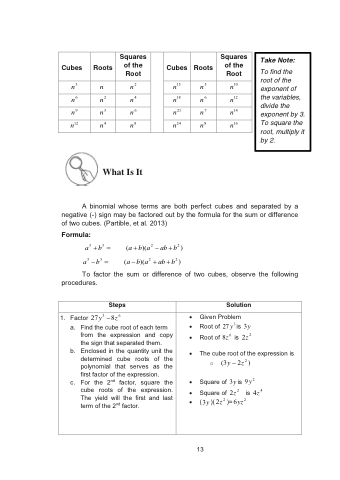
Squares
of the
Cubes
Roots
Root
Cubes
Roots
Squares
of the
Take Note:
To find the root of the exponent of the variables, divide the exponent by 3. To square the root, multiply it by 2.
n3
n6
n9
n12
n
n2
n3
n4
n2
n4
n6
n8
n15
n18
n21
n24
n5
n6
n7
n8
Root
n10
n12
n14
n16
What Is It
a3 b3  (ab)(a2 abb2) a3 b3  (ab)(a2 abb2)
A binomial whose terms are both perfect cubes and separated by a
negative (-) sign may be factored out by the formula for the sum or difference
of two cubes. (Partible, et al. 2013)
Formula:
To factor the sum or difference of two cubes, observe the following
procedures.
Steps
 
 

 
o
Solution
1.
a.
b.
c.
Factor 27y3 8z6
Find the cube root of each term
from the expression and copy
the sign that separated them.
Enclosed in the quantity unit the
The cube root of the expression is
determined cube roots of the
(3y  2z2 )
polynomial that serves as the
first factor of the expression.
Squareof 3yis 9y2
For the 2nd factor, square the
cube roots of the expression.
Square of 2z2 is 4z4
The yield will the first and last
(3y)(2z2 )=6yz2
term of the 2nd factor.
Given Problem
Rootof 27y3is 3y
Rootof8z6 is2z2
13