Page 36 - Nicolaes Witsen & Shipbuilding in the Dutch Golden Age

Chapter 1
board and on a theoretical basis, which produced a mani- fold increase in the rate of change. At the beginning of the eighteenth century the Amsterdam Admir alty even fou nd itself forced to impor t foreign exper tise to moderniz e its shipbuilding techniques, and English shipwrights were appointed to the Amsterdam Admiralty.24
Although nothing points to the use of mathematical practices on the yards, Witsen himself insists on demon- strating such a method to obt ain the sh ape of the m ain frame (see fig. 1.11).
We find no trace of the use ofsuch geometrical methods in contemporary sources. In contracts and descriptions of ships of that era there is never any mention of the lengths of sweeps or the locations of the centers from whence the sweeps had to be struck. And if applied to Witsen’s pinas, the method produc es a c ompletely different shape (see fig. 1.12). In a ll probability it was Witsen himself, aware of the geometrical methods applied in other Europe an coun- tries, who devised this method, either to raise the status of the building method he adver tised, or from a need to fi nd explanations for the phenomena he encountered.
Even the sober Van Yk, when de aling with the sh ape of the (m ain) frames, writes: “Wh at figure these fr ames are to take, depends largely on the width of the ship, and the depth, and fur ther on the eye and the judgment of the master shipbuilder. But the shallowness of the Dutch estuaries is often the reason that he cannot take into ac- count that, which otherwise he would think best” (p. 69). Concerning the fore and after body , he st ates: “I h ave never found that our shipbuil ders have any secure, or fixed rules regarding the shaping of these frames” (p. 76).
If Witsen’s geometrical method was used by shipbuild- ers after all, it can only have been to des ign a frame on paper. In the yard it would have been useless, for with the shell-first method, no entire frame was ever assembled to be raised on the k eel; instead, section af ter section was fitted in the hull, following the building stages.
Ship Measurement
18
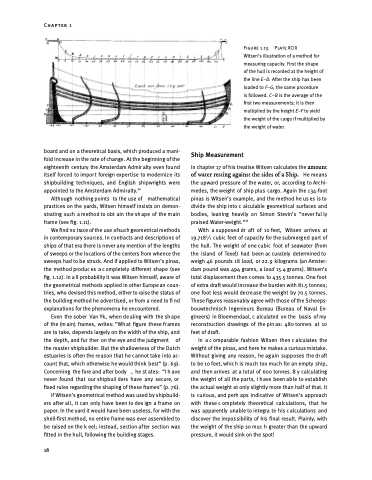
Figure 1.13. Plate XCIII
Witsen’s illustration of a method for measuring capacity. First the shape
of the hull is recorded at the height of the line E–D. After the ship has been loaded to F–G, the same procedure
is followed. C–B is the average of the first two measurements; it is then multiplied by the height E–F to yield the weight of the cargo if multiplied by the weight of water.
In chapter 17 of his treatise Witsen calculates the amount of water resting against the sides of a Ship. He means the upward pressure of the w ater, or, according to Arc hi- medes, the weight of ship plus cargo. Again the 134-foot pinas is Witsen’s example, and the method he us es is to divide the ship into c alculable geometrical surfaces and bodies, leaning heavily on Simon Stevin’s “never ful ly praised Water-weight.”25
With a supposed dr aft of 10 feet, Witsen arrives at 19.7181⁄3 cubic feet of capacity for the submerg ed part of the hull. The weight of one cubic foot of seawater (from the island of Texel) had been ac curately determined to weigh 46 pounds 18 lood, or 22.9 kilograms (an Amster- dam pound was 494 grams, a lood 15.4 grams). Witsen’s total displacement then comes to 435.5 tonnes. One foot of extra draft would increase the burden with 81.5 tonnes; one foot less would decrease the weight by 70.5 tonnes. These figures reasonably agree with those of the Scheeps- bouwtechnisch Ingenieurs Bureau (Bureau of Naval En- gineers) in Bloemendaal, c alculated on the basis of my reconstruction drawings of the pin as: 480 tonnes at 10 feet of draft.
In a c omparable fashion Witsen then c alculates the weight of the pinas, and here he makes a curious mistake. Without giving any reason, he ag ain supposes the dr aft to be 10 feet, whic h is much too much for an empty ship, and then arrives at a tot al of 600 tonnes. B y calculating the weight of all the par ts, I h ave been ab le to est ablish the actual weight at only slightly more than half of that. It is curious, and perh aps indicative of Witsen’s approach with these c ompletely theoretical calculations, that he was apparently unable to integra te his c alculations and discover the impossibility of his final result. Plainly, with the weight of the ship so muc h greater than the upward pressure, it would sink on the spot!