Page 62 - Maxwell House

42 Chapter 1

Integral Form Differential Form

8 = =

9 = =
0
0
We now placed in all equations the excitation charges
0 and currents discussed in the previous section. The
constituency equations for these sources are not included
because, as it has been mention before, the nature of them
q can be very complicated and they could not follow
qv Maxwell’s equations. As we will demonstrate in Chapter
2, in case of monochromatic field the material
parameters , , and can be the complex numbers

having the real and imaginary parts while the imaginary
part is responsible for the energy loss in materials.
0
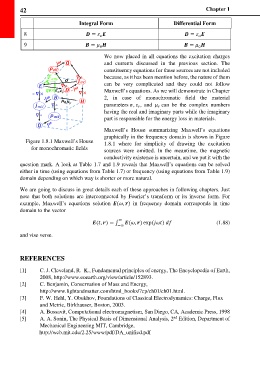
Maxwell’s House summarizing Maxwell’s equations
graphically in the frequency domain is shown in Figure
Figure 1.8.1 Maxwell’s House 1.8.1 where for simplicity of drawing the excitation
for monochromatic fields sources were omitted. In the meantime, the magnetic
conductivity existence is uncertain, and we put it with the
question mark. A look at Table 1.7 and 1.9 reveals that Maxwell’s equations can be solved
either in time (using equations from Table 1.7) or frequency (using equations from Table 1.9)
domain depending on which way is shorter or more natural.

We are going to discuss in great details each of these approaches in following chapters. Just
note that both solutions are interconnected by Fourier’s transform or its inverse form. For
example, Maxwell’s equations solution (, ) in frequency domain corresponds in time
domain to the vector

∞
(, ) = ∫ (, ) exp() (1.88)
−∞
and vise verse.

REFERENCES

[1] C. J. Cleveland, R. K., Fundamental principles of energy, The Encyclopedia of Earth,
2008, http://www.eoearth.org/view/article/152893.
[2] C. Benjamin, Conservation of Mass and Energy,
http://www.lightandmatter.com/html_books/7cp/ch01/ch01.html.
[3] F. W. Hehl, Y. Obukhov, Foundations of Classical Electrodynamics: Charge, Flux
and Metric, Birkhauser, Boston, 2003.
[4] A. Bossavit, Computational electromagnetism, San Diego, CA, Academic Press, 1998
[5] A. A. Sonin, The Physical Basis of Dimensional Analysis, 2 Edition, Department of
nd
Mechanical Engineering MIT, Cambridge,
http://web.mit.edu/2.25/www/pdf/DA_unified.pdf