Page 102 - Mechatronics with Experiments

88 MECHATRONICS
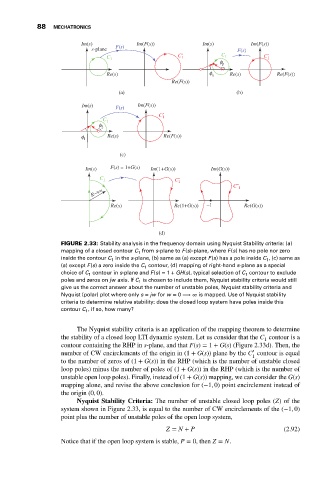
Im(s) Im(F(s)) Im(s) Im(F(s))
s-plane F(s) F(s)
C 1 C´ 1 C 1 C´ 1
ϕ p
Re(s) ϕ x Re(s) Re(F(s))
Re(F(s))
(a) (b)

Im(s) F(s) Im(F(s))
C´ 1
C 1
ϕ 2
ϕ 1 Re(s) Re(F(s))

(c)

Im(s) F(s) = 1+G(s) Im(1+G(s)) Im(G(s))
C 1 C´ 1
C˝ 1
R→∞

Re(s) Re(1+G(s)) –1 Re(G(s))

(d)
FIGURE 2.33: Stability analysis in the frequency domain using Nyquist Stability criteria: (a)
mapping of a closed contour C 1 from s-plane to F(s)-plane, where F(s) has no pole nor zero
inside the contour C in the s-plane, (b) same as (a) except F(s) has a pole inside C , (c) same as
1
1
(a) except F(s)azero insidethe C 1 contour, (d) mapping of right-hand s-plane as a special
choice of C contour in s-plane and F(s) = 1 + GH(s), typical selection of C contour to exclude
1
1
poles and zeros on jw axis. If C is chosen to include them, Nyquist stability criteria would still
1
give us the correct answer about the number of unstable poles, Nyquist stability criteria and
Nyquist (polar) plot where only s = jw for w = 0 ⟶ ∞ is mapped. Use of Nyquist stability
criteria to determine relative stability: does the closed loop system have poles inside this
, if so, how many?
contour C 1
The Nyquist stability criteria is an application of the mapping theorem to determine
the stability of a closed loop LTI dynamic system. Let us consider that the C contour is a
1
contour containing the RHP in s-plane, and that F(s) = 1 + G(s) (Figure 2.33d). Then, the
′
number of CW encirclements of the origin in (1 + G(s)) plane by the C contour is equal
1
to the number of zeros of (1 + G(s)) in the RHP (which is the number of unstable closed
loop poles) minus the number of poles of (1 + G(s)) in the RHP (which is the number of
unstable open loop poles). Finally, instead of (1 + G(s)) mapping, we can consider the G(s)
mapping alone, and revise the above conclusion for (−1, 0) point encirclement instead of
the origin (0, 0).
Nyquist Stability Criteria: The number of unstable closed loop poles (Z)ofthe
system shown in Figure 2.33, is equal to the number of CW encirclements of the (−1, 0)
point plus the number of unstable poles of the open loop system,

Z = N + P (2.92)
Notice that if the open loop system is stable, P = 0, then Z = N.