Page 24 - modul trigonometri bilingual

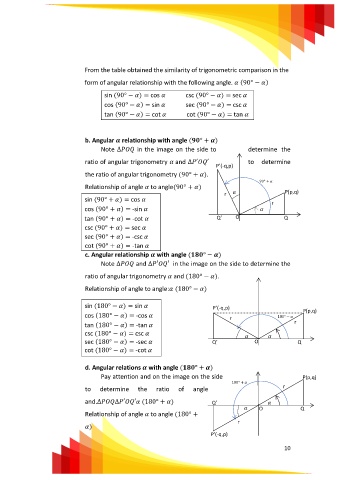
From the table obtained the similarity of trigonometric comparison in the
form of angular relationship with the following angle. (90° − )

sin (90° − ) = cos csc (90° − ) = sec

cos (90° − ) = sin sec (90° − ) = csc
tan (90° − ) = cot cot (90° − ) = tan

b. Angular relationship with angle ( ° + )
Note ∆ in the image on the side to determine the

ratio of angular trigonometry and ∆ ′ ′ to determine
P’(-q,p)
the ratio of angular trigonometry (90° + ).
90° +
Relationship of angle to angle(90° + )
r P(p,q)
sin (90° + ) = cos
r
cos (90° + ) = -sin
tan (90° + ) = -cot Q’ O Q
csc (90° + ) = sec
sec (90° + ) = -csc
cot (90° + ) = -tan
c. Angular relationship with angle ( ° − )
Note ∆ and ∆ ′ ′ in the image on the side to determine the

ratio of angular trigonometry and (180° − ).

Relationship of angle to angle: (180° − )

sin (180° − ) = sin P’(-q,p)
P(p,q)
cos (180° − ) = -cos r 180° −
tan (180° − ) = -tan r
csc (180° − ) = csc
sec (180° − ) = -sec Q’ O Q
cot (180° − ) = -cot

d. Angular relations with angle ( ° + )
Pay attention and on the image on the side P(p,q)
180° +
to determine the ratio of angle r
and.∆ ∆ ′ ′ (180° + ) Q’
O Q
Relationship of angle to angle (180° +
r
)
P’(-q,p)

10