Page 148 - classs 6 a_Neat

CONSTRUCTION OF SOME SPECIAL ANGLES USING A PAIR OF COMPASSES EXERCISE 9-A

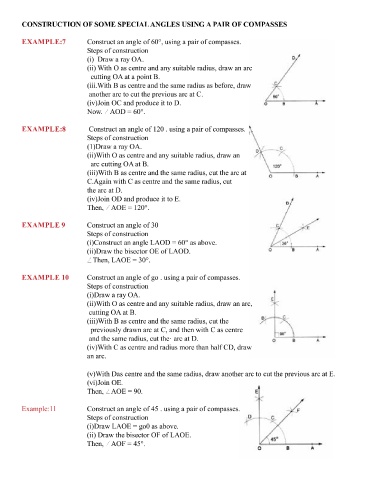
EXAMPLE:7 Construct an angle of 60°, using a pair of compasses. 1. Using a ruler and a compass, draw line segments of the following lengths.
Steps of construction a.5.5 cm b.7.2 cm c.4.8 cm d.6.4 cm e.3.6 cm
(i) Draw a ray OA. 2. Draw two line segments AB = 2.5 cm and CD = 4.4 cm. Construct the line segments of the following lengths.
(ii) With O as centre and any suitable radius, draw an arc a.AB+ CD b.CD -AB c.2AB 1 CD e.3AB -CD
cutting OA at a point B. 2
(iii.With B as centre and the same radius as before, draw 3. Draw a line segment of length 4.5 cm. Mark a point Pon it. Construct a perpendicular to the line segment
another arc to cut the previous arc at C. passing through the point P.
(iv)Join OC and produce it to D. 4. Draw a line segment of length 6.6 cm and construct its perpendicular bisector.
Now. AOD = 60°. 5. Draw a line segment of length 5.5 cm. Draw a perpendicular to the line from point Plying outside the line.
6. Measure the following line segments using a divider and then list them in ascending order.
EXAMPLE:8 Construct an angle of 120 . using a pair of compasses.
Steps of construction a. A B b.
(1)Draw a ray OA. Y
(ii)With O as centre and any suitable radius, draw an
arc cutting OA at B. c. P X
(iii)With B as centre and the same radius, cut the arc at
C.Again with C as centre and the same radius, cut Q
the arc at D.
(iv)Join OD and produce it to E. 7. Draw a circle of radius 4.5 cm with centre at 0. Mark three points P, Q and R such that P is on the circle, Q is
Then, AOE = 120°. in the interior of the circle and R is in the exterior of the circle.

EXAMPLE 9 Construct an angle of 30 8. Draw three circles with the same centre and radii of 3 cm, 3.5 cm and 4 cm, respectively. What are these
Steps of construction circles called ?
(i)Construct an angle LAOD = 60° as above.
(ii)Draw the bisector OE of LAOD. 9. Draw a line segment AB = 5 cm. Find its perpendicular bisector, which will give the midpoint of AB.
Then, LAOE = 30°. Mark it as 0. With Oas the centre and OA or OB as the radii, draw a circle. What is the line segment AOB called

in relation to the circle?
EXAMPLE 10 Construct an angle of go . using a pair of compasses.
Steps of construction 10. Draw a circle with centre A and radius 3.5 cm. Mark a point B on the circumfer ence. Now with Bas
(i)Draw a ray OA. the centre and radius 3.5 cm, draw another circle. Does it pass through A? Join the centres A and B
(ii)With O as centre and any suitable radius, draw an arc, and find its perpendicular bisector.
cutting OA at B. EXERCISE 9-B
(iii)With B as centre and the same radius, cut the
previously drawn arc at C, and then with C as centre 1. Use a protractor and draw the following angles.
and the same radius, cut the· arc at D. a. 75° b. 100° c. 140°
(iv)With C as centre and radius more than half CD, draw
an arc. 2. Draw an angle of 135° with the help of a protractor and bisect it using a compass.
3. Draw an angle ∠ABC = 70° with the help of a protractor. Now, draw ∠ PQR=∠ABC using a ruler and a
(v)With Das centre and the same radius, draw another arc to cut the previous arc at E. compass.
(vi)Join OE. 4. Using a ruler and a compass, construct an angle of 60°. Bisect the angle and then construct an angle of 30°
Then, AOE = 90. 5. Using a ruler and a compass, construct an angle of 120°.
6. Construct an angle of 45° using a ruler and a compass. Also bisect the angle.
Example:11 Construct an angle of 45 . using a pair of compasses. 7. Draw LPQR = 65°. Using a protractor, bisect the angle. Take a point K on the angular bisector and draw per-
Steps of construction pendiculars on the arms PQ and QR.
(i)Draw LAOE = go0 as above. 1
(ii) Draw the bisector OF of LAOE. 8. Draw ∠AOB = 100° and construct ∠ XYZ such that ∠ XYZ = ∠ AOB.
4
Then, AOF = 45°.